Parabola, Graphing Vertex and XIntercepts Root plot for y = x 24x12 Axis of Symmetry (dashed) {x}={ 0} Vertex at {x,y} = { 0,1600} x Intercepts (Roots) Root 1 at {x,y} = {0, 000} Root 2 at {x,y} = { 600, 000} Solve Quadratic Equation by Completing The Square 52 Solving x 24x12 = 0 by Completing The SquareAnswer Answer The required xintercepts are (4, 0) and (7, 0) Stepbystep explanation We are given to find the xintercepts for the parabola defined by the following equation the xintercepts of a function are the points where the y coordinate is zero Therefore, the xintercepts of the given function are (4, 0) and (7, 0)Parabola, Graphing Vertex and XIntercepts Root plot for y = x 2 4x12 Axis of Symmetry (dashed) {x}={0} Vertex at {x,y} = {0,1600} x Intercepts (Roots) Root 1 at {x,y} = {600, 000} Root 2 at {x,y} = { 0, 000} Solve Quadratic Equation by Completing The Square 32 Solving x 2 4x12 = 0 by Completing The Square
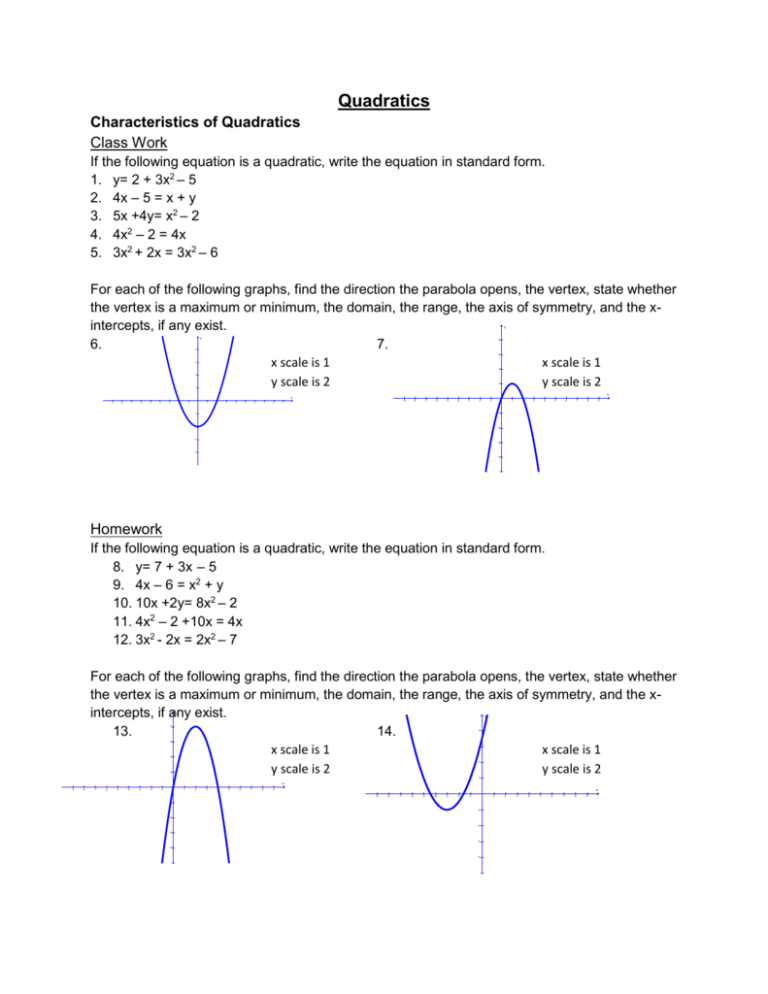
Quadratics
X intercept of y=x^2-4x-12
X intercept of y=x^2-4x-12-Root Factored Form one x intercept If a parabola cuts the x axis in one point ( p, 0) then the parabola 's equation, y = a x 2 b x c, can be written y = a ( x − p) 2 where p is the x coordinate of the point at which the parabola cuts the x axis (the x intercept)Solution Steps y = x2 y = x − 2 Swap sides so that all variable terms are on the left hand side Swap sides so that all variable terms are on the left hand side x2=y x − 2 = y Add 2 to both sides Add 2 to both sides




3 For Y X2 4x 12 0 Find The Y Intercept Chegg Com
Solve Quadratic Equation by Completing The Square 22 Solving x24x12 = 0 by Completing The Square Subtract 12 from both side of the equation x24x = 12 Now the clever bit Take the coefficient of x , which is 4 , divide by two, giving 2 , and finally square it giving 4 Add 4 to both sides of the equation On the right hand side we haveQuestion Find the xintercepts y = x2 3x – 10 Answer by jim_thompson5910() (Show Source) You can put this solution on YOUR website!The yintercept is the value of y when x = 0 to find that, simply replace x with 0 in your equation to get y = x^2 4x 3 becomes y = 0 0 3 which becomes y = 3 as confirmed by the graph to plot the graph, you simply take values of x and solve for y and then plot the value of the (x
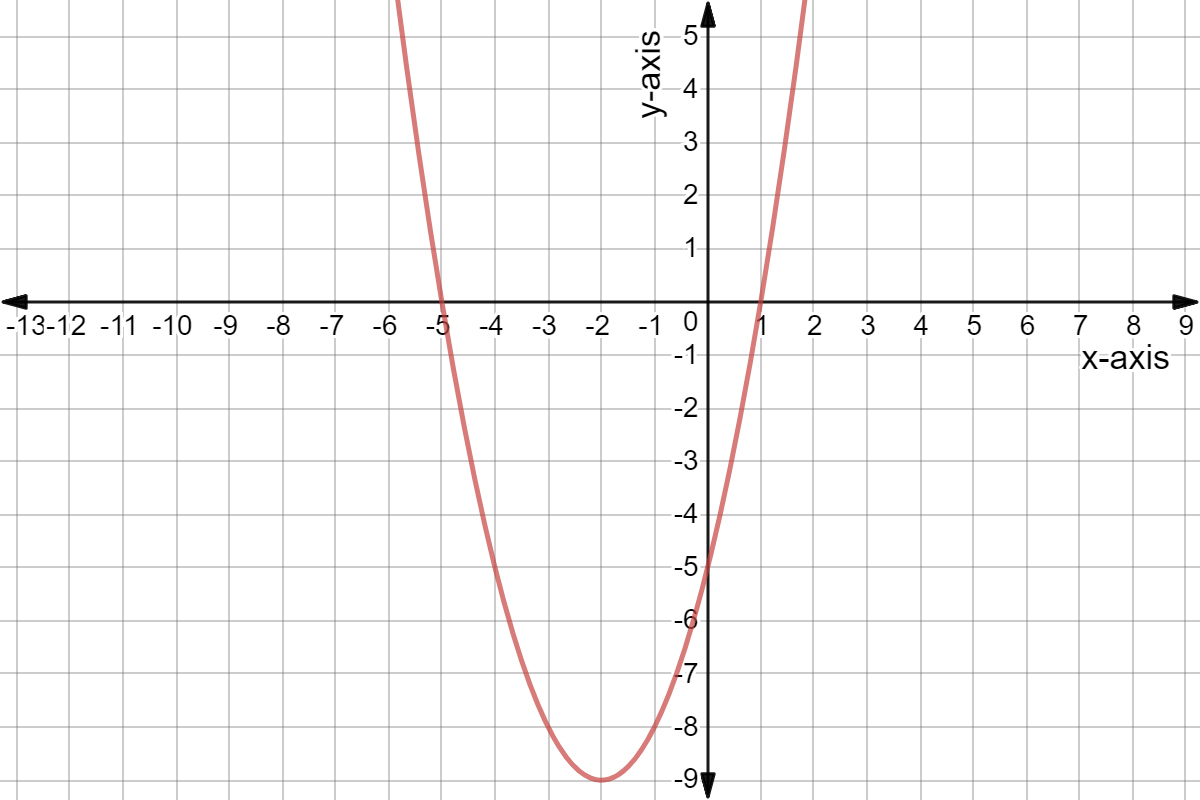
Graph the equation \(y=x^24x12\) by finding the vertex, the \(x\) and \(y\)intercepts, and any additional points necessary to make a nice parabola First, notice that \(a=1\text{,}\) which is more than zero, so we know the parabola will be opening upwardAlgebra Find the Vertex y=x^24x12 y = x2 − 4x − 12 y = x 2 4 x 12 Rewrite the equation in vertex form Tap for more steps Complete the square for x 2 − 4 x − 12 x 2 4 x 12 Tap for more steps Use the form a x 2 b x c a x 2 b x c, to find the values of a a, b b, and c c a = 1, b = − 4, c = − 12 a = 1, bFind the intercepts for the quadratic equation y = x2−4x−12 y = x 2 − 4 x − 12 using algebra To determine the y y intercept, we substitute x =0 x = 0 and find y= 02−4(0)−12 = −12 y = 0 2 − 4 ( 0) − 12 = − 12 So the y y intercept occurs where y = −12 y = − 12 On a graph, this is the point (0,−12) ( 0, − 12)
F(x) = x² x 2 Equation 1 Firstly let's plot the Graph of Equation 1 Graph 1 EQUATION 1 Let us also plot Graph 2 below which is a higher resolution image of the intercept X intercept means the point at which the line or the curve that intersects xaxis, and y intercept is the intersection at yaxis For the y intercept, we know at yaxis, x=0 SO, y =0^2 4*0 12 = 12 So 12 is the yintercept, For the xintercept, we let y=0 x^2 4x12=0 x^2 (62) 12=0 x^2 6x 2x 12=0 x(x6) 2(x6)=0 (x6)(x2) =0 So either Or x6=0 x2 =0 x=6 x=2 So 6 and 2 are the xinterceptsTo find the xintercept, set and solve for (,)



What Are The X Intercepts Of Y X 2 6x 4 Quora



1
Solve your math problems using our free math solver with stepbystep solutions Our math solver supports basic math, prealgebra, algebra, trigonometry, calculus and more y = x² 4x 12 When y = 0 x² 4x 12 = 0 x² 4x 12 = 0 (x 2)(x 6) = 0 x = 2 or x = 6 The xintercepts are 2 and 6 y = (x² 4x) 12 y = (x²Find the X and Y Intercepts y=x^24x12 Find the xintercepts Tap for more steps To find the xintercept (s), substitute in for and solve for Solve the equation Tap for more steps Rewrite the equation as Factor using the AC method Tap for more steps Consider the form



Eq How Do I Find The X And Y Intercepts Of Linear Equations Ppt Download



2 4 Grapgs Of Second Degree Functions
Explanation \displaystyle{y}={x}^{{2}}{4}{x}{12}{\quad\text{or}\quad}{y}={x}^{{2}}{4}{x}{4}{8} Vertex is at ( 2 , 8 ) , y intercept is y = 1 2 or at ( 0 , 1 2 ) , xintercept is absent Explanation y = x 2 − 4 x 1 2 or y = x 2 − 4 x 4 8For any parabola , the xcoordinate of the vertex is at Calculate that value, substitute it for x, and then calculate the ycoordinate value The xintercepts are ordered pairs of the form (x,0), So set the trinomial equal to zero and solve, either by factoring and using the zero productWhat are the y and the x intercepts of this parabola f(x) =x^2x2?




Quadratics



X Intercepts And Y Intercepts Of A Functions And Finding Them Example 2 Youtube
Lets find out the vertex and the x intercepts To find out the vertex we use formula The value of a= 1, b=4 Plug in the values Substitute x=2 and find out y So vertex (2,25) Now we find out x intercepts, replace y with 0 and solve for x we find out two factors whose product 21 and add upto 4 the factors are 7 and 3 x intercepts are 3,7Set the right side equal to zero Notice we have a quadratic equation in the form of where , , and Let's use the quadratic formula to solve for x y^2= 2/3y5/4 are there two real solutions, 2 nonreal solutions or one real soltion?



Answered Find The Two X Intercepts Of The Bartleby



Solution Find The X Intercepts Y X 2 4x 32
Let R be the region bounded by the graphs of y=cos((pi x)/2) and y=x^2(26/5)x1 A Find the area of R B The vertical line x=k splits the region R into two equal parts Write, but do not solve, an equation involving integrals You can view more similar questions or ask aThe yintercept is (0, 12) The xintercept of a equation is the location where the graph crosses the xaxis To find the xintercept of an equation, substitute y = 0 into the equation and solve for x by factoring or using the quadratic formula Here are two examples Find the xintercept for the equation y = x2 4x 12 If y = 0, thenReview Vertex and Intercepts of a Quadratic Functions The graph of a quadratic function of the form f(x) = a x 2 b x c is a vertical parabola with axis of symmetry parallel to the y axis and has a vertex V with coordinates (h , k), x intercepts when they exist and a y intercept as shown below in the graph When the coefficient a is positive the vertex is the lowest point in the



Solution How Do You Find The X Intercept Of The Equation Y X 2 4x 12



Http Www Eastauroraschools Org Cms Lib Ny Centricity Domain 323 Ch 8 review sheet answers Pdf
Determine the concavity, the xintercepts and the yintercept of the parabola whose equation is y = x^2 4x 12 Write the equation y = x^2 4x 12 in vertex form Sketch the graph of the equation y = x^2 4x 12Get stepbystep solutions from expert tutors as fast as 1530 minutes Your first 5 questions are on us!Question What are the intercepts for the equation y=x^24x4 Found 3 solutions by Fombitz, checkley79, ewatrrr Answer by Fombitz() (Show Source) You can put this solution on YOUR website!



Ppt 1 6 Powerpoint Presentation Free Download Id



Http Www Manhassetschools Org Cms Lib8 Ny Centricity Domain 710 Group work 59 63 solutions Pdf
So, replace x with 0 to get y = 0 2 − 4 ( 0) − 12 Evaluate y = − 12 So, the yintercept is 12 (c) Coordinates of the vertex Take y = x² − 4x − 12 Rewrite as y = x² − 4x 4 − 12 4 the net effect of adding 4 and subtracting 4 is ZERO Rewrite as y = (x² − 4x 4) − 16 Factor to get y = (x 2)² − 16Rewrite to Intercept Form y = x2 – 4x – 12 Intercept form_____ Identify the xintercepts ( , ) and ( , ) Rewrite to Vertex Form y = x2 – 4x Graph y = x2 – 4x – 12 You must include 5 distinct points Be sure to study all information regarding unit 5! Find area of the isosceles triangle formed by the vertex and the xintercepts of parabola y=x^24x−12 Guest 0 users composing answers 1 0 Answers #1 284 1 To find the xintercepts y = x 2 4x 12 0 = x 2 4x 12 0 = (x 6)(x 2) xintercepts x = 6 and x = 2 To find the




7 3 Linear Equations And Their Graphs Objective To Graph Linear Equations Using The X And Y Intercepts To Graph Horizontal And Vertical Lines Ppt Download




Identifying The Intercepts On The Graph Of A Line Prealgebra
Solve your math problems using our free math solver with stepbystep solutions Our math solver supports basic math, prealgebra, algebra, trigonometry, calculus and moreY=x^24x12 Answer by solver () ( Show Source ) You can put this solution on YOUR website!Equation of a Line Given Points;




Using X Intercepts To Graph Polynomial Functions Study Com



For The Quadratic Function F X X2 4x 12 Chegg Com
Y=x^{2}4x12 en Related Symbolab blog posts Functions A function basically relates an input to an output, there's an input, a relationshipTo find the xintercept (s), substitute in 0 0 for y y and solve for x x 0 = x 2 − 9 x 0 = x 2 9 x Solve the equation Tap for more steps Rewrite the equation as x 2 − 9 x = 0 x 2 9 x = 0 x 2 − 9 x = 0 x 2 9 x = 0 Factor x 2 − 9 x x 2 9 xDirectrix y = −65 4 y = 65 4 Select a few x x values, and plug them into the equation to find the corresponding y y values The x x values should be selected around the vertex Tap for more steps Replace the variable x x with 1 1 in the expression f ( 1) = ( 1) 2 − 4 ⋅ 1 − 12 f ( 1) = ( 1) 2 4 ⋅ 1 12 Simplify the result



Chapter 2 Functions And Linear Equations Functions Vs Relations A Relation Is Just A Relationship Between Sets Of Information A Function Is A Well Behaved Ppt Download




Graphing Parabolas
The material will beThe intercepts of a graph are points at which the graph crosses the axes The xintercept is the point at which the graph crosses the xaxisAt this point, the ycoordinate is zeroThe yintercept is the point at which the graph crosses the yaxisAt this point, the xcoordinate is zero To determine the xintercept, we set y equal to zero and solve for xFunctions Is a Function;




Graph Quadratic Functions Using Properties Intermediate Algebra
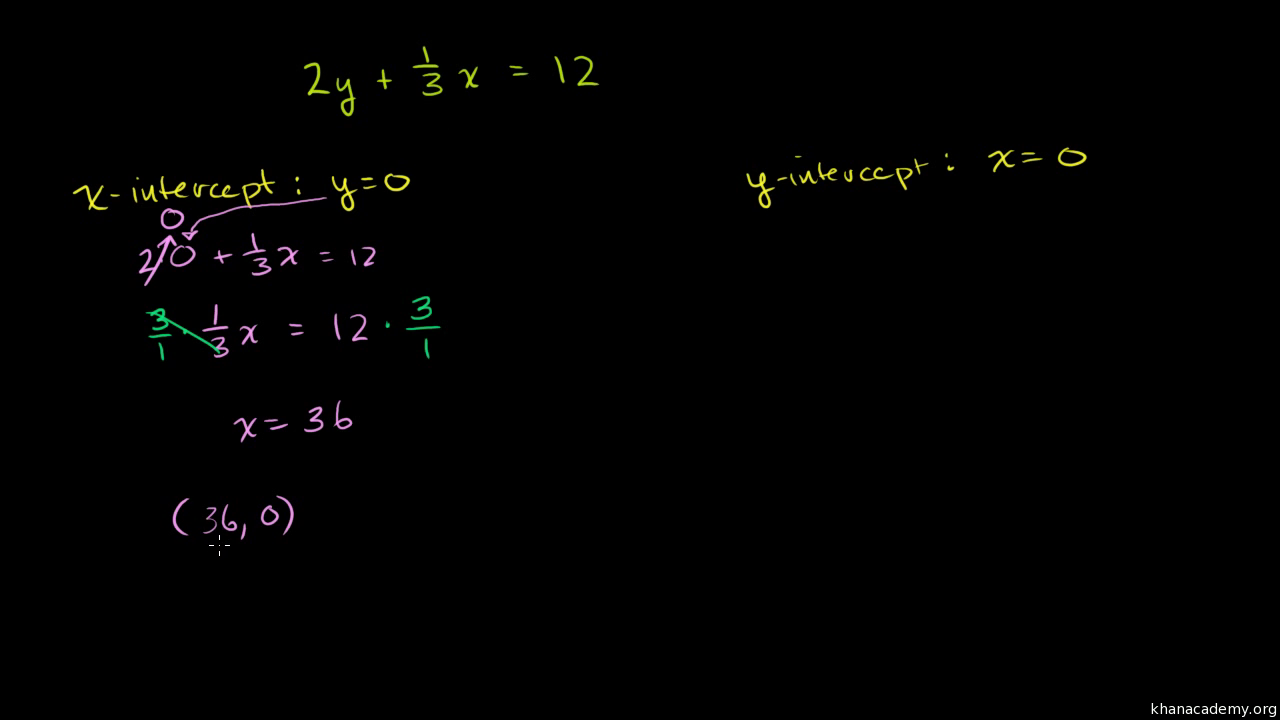



Worked Example Intercepts From An Equation Video Khan Academy
Rewrite to Intercept Form y = x 2 – 4x – 12 Intercept form_____ Identify the xintercepts ( , ) and ( , ) Rewrite to Vertex Form y = x – 4x – 12 Graph y = x2 – 4x – 12 You must include 5 distinct points Be sure to study all information regarding unit 5! We know that the xintercepts are 2 and 6 So, halfway between them on the x line is positive 2 Therefore x=2 at the vertex Plug that into the equation y=x^24x12 and solve for y I know this wont work for every question of this type but it might save some time in this particular question • Comment x = 2 2√2 i and 2 2√2 i Stepbystep explanation Given the function, y=x^24x12 x intercept occurs at y = 0 Substitute y = 0 0=x^24x12 x^2 4x 12 = 0 Factorize x = 4±√4²4(12)/2 x = 4±√1648/2 x = 4±√32/2 x = 4 ± √32 i/2 x = 44√2 i/2 and x =44√2 i/2 x = 2 2√2 i and 2 2√2 i




Find Intercepts And Slope Of Linear Equation 3y 4x 12 Youtube
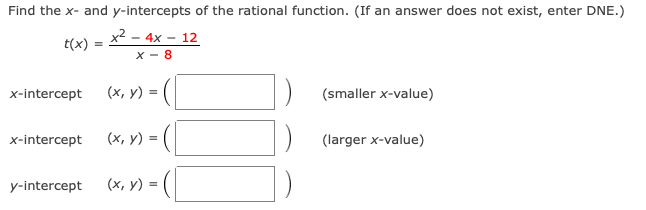



Answered Find The X And Y Intercepts Of The Bartleby
Start Point (new) End Point (new) Parallel;Solve your math problems using our free math solver with stepbystep solutions Our math solver supports basic math, prealgebra, algebra, trigonometry, calculus and more Find the vertex and intercepts for each quadratic function and SKETCH ITS GRAPH #49 y = x^2 4x 12 #50 y= x^2 2x 24 Answered by a verified Tutor We use cookies to give you the best possible experience on our website



How Do You Write F X 2x 2 12x 12 In Vertex Form Socratic



Graphing Quadratic Functions
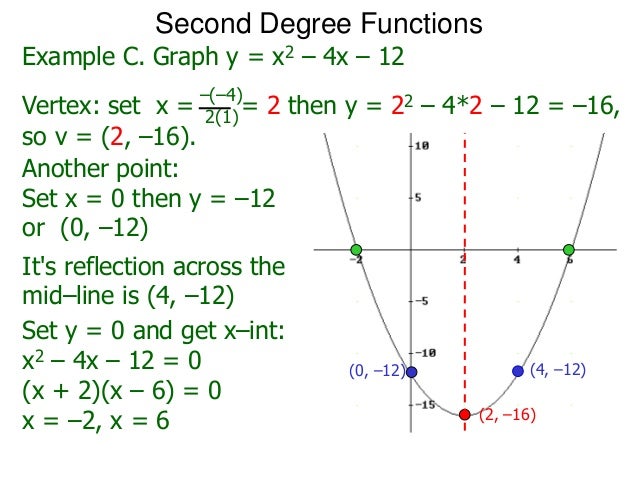
Find the x and y intercepts x^24x12 open a book to two facing pages the product of teh page is 930 wht are the page numbers solve for given variable assume vairable represent nonnegative numbers A=7s for s give exact solutions to three decimal places (x8)^2=18 farmer planting aFor the parabola y = x^2 4x 12 in the xyplane, find the following (a) The xintercepts (b) The yintercept (c) Coordinates of the vertex a x = 6 , x = 2 b 12 c x = (b/2a , y ) = (2 , 16) For the circle ( x 1 )^2 ( y 1 )^2 = in the xyplane, find the following71 Find the Vertex of y = x 24x12 Parabolas have a highest or a lowest point called the Vertex Our parabola opens up and accordingly has a lowest point (AKA absolute minimum) We know this even before plotting "y" because the coefficient of the first term, 1 , is positive (greater than zero)




Solved Find The Intercepts Of The Function F X F X X Chegg Com



Www Scasd Org Cms Lib Pa Centricity Domain 1229 2 2 p2 unit 2 2 note packet lg 1019 complete lt 8 to end Pdf
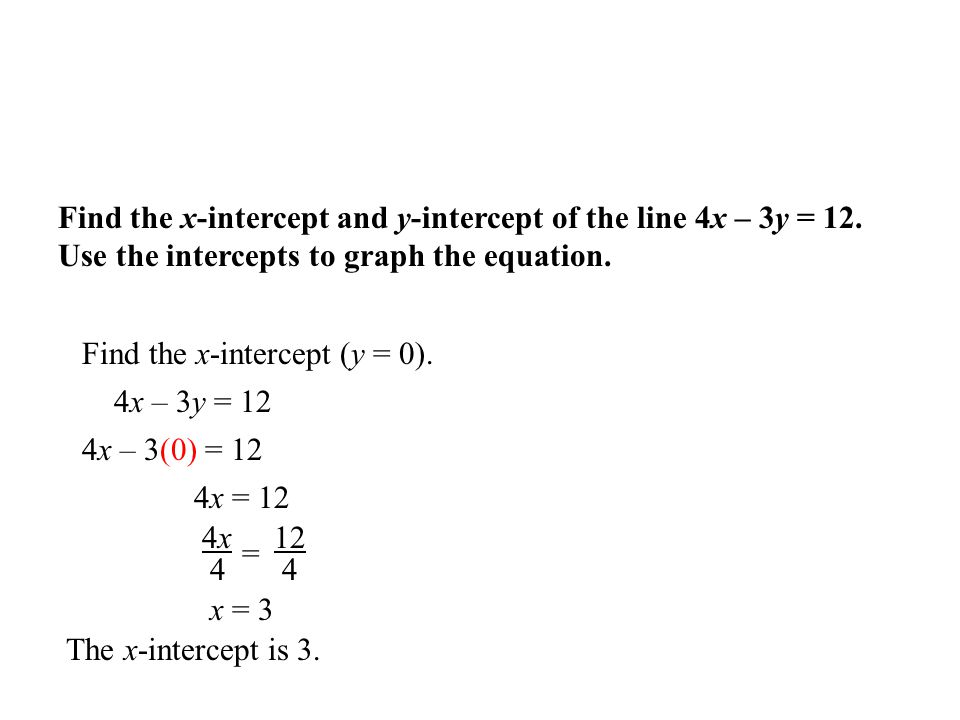
The material will beGiven Slope & Point;Calculate the XIntercept When y = 0 the value of x is 1/2 Our line therefore "cuts" the x axis at x= xintercept = 2/4 = 1/2 = Calculate the Slope Slope is defined as the change in y divided by the change in x We note that for x=0, the value of y is 00 and for x=00, the value of y is



Solved Please Show Work I Already Have The Correct Answer Chegg Com




Which Of The Following Graphs Is Described By The Function Given Below Y X 2 4x 12 Brainly Com
x and y intercepts for y=x^24x12 Find the x and y intercepts for the equation Follow Hi Zach Since y^2 = x − 2 is a relation (has more than 1 yvalue for each xvalue) and not a function (which has a maximum of 1 yvalue for each xvalue), we need to split it into 2 separate functions and graph them together So the first one will be y 1 = √ (x − 2) and the second one is y 2 = −√ (x − 2)




Graph The Parabola And Give Its Vertex Axis X Intercepts And Y Intercept Y 2x 2 8x 16 Study Com



Systems Of Linear Equations



Unique Quadratic Equation In The Form Y Ax 2 Bx C




Worked Example Intercepts From An Equation Video Khan Academy




Graphing Parabolas




Intercepts Of The Function F X F X X 3 3x 2 Chegg Com




Answered Find The Two X Intercepts Of The Bartleby




Answered For The Quadratic Function F X Bartleby



Pcc Instructure Com Courses Files Download Download Frd 1




Plot The X Intercept S Y Intercept Vertex And Axis Of Symmetry For The Function Below G X Brainly Com
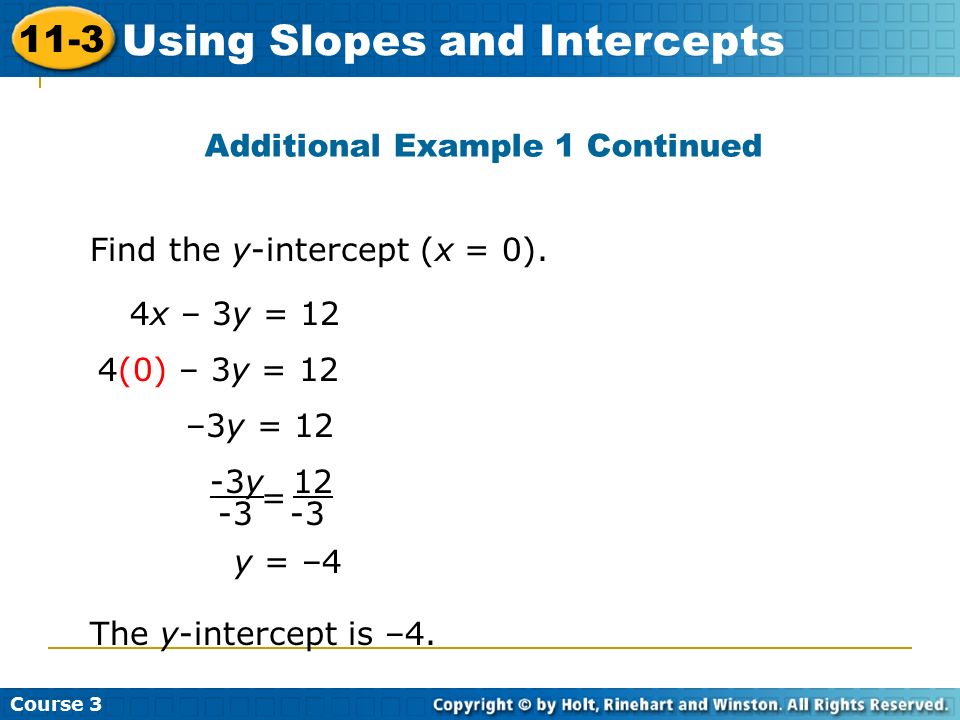



Vocabulary X Intercept Y Intercept Slope Intercept Form Insert Lesson Title Here Course Using Slopes And Intercepts Ppt Download




Graphs To Solve Quadratic Equations Ck 12 Foundation



Y X 2 12x 35 Math Homework Answers



Q Tbn And9gcqrwbw8rwgn5klaw Psm3hacdylhgqiipgixekcm2iyng77sxm1 Usqp Cau




Graphing Parabolas



Unique Quadratic Equation In The Form Y Ax 2 Bx C



Unique Quadratic Equation In The Form Y Ax 2 Bx C
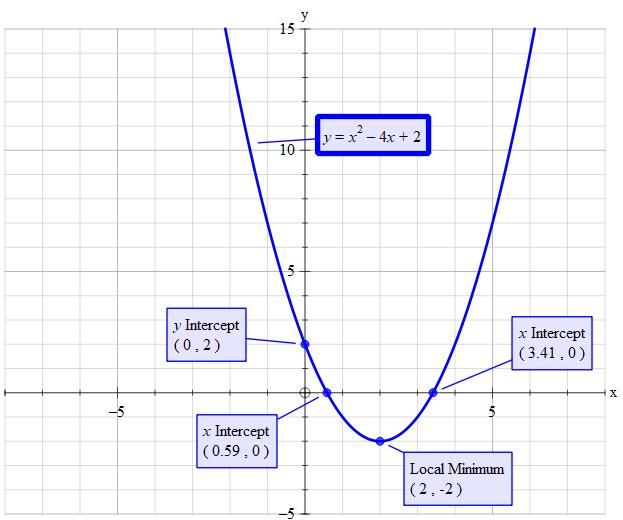



How Do You Identify The Important Parts Of Y X 2 4x 2 To Graph It Socratic




A Sketch The Graph Of The Function Y X 2 4x B Find Its Vertex Y Intercept And X Intercepts Study Com
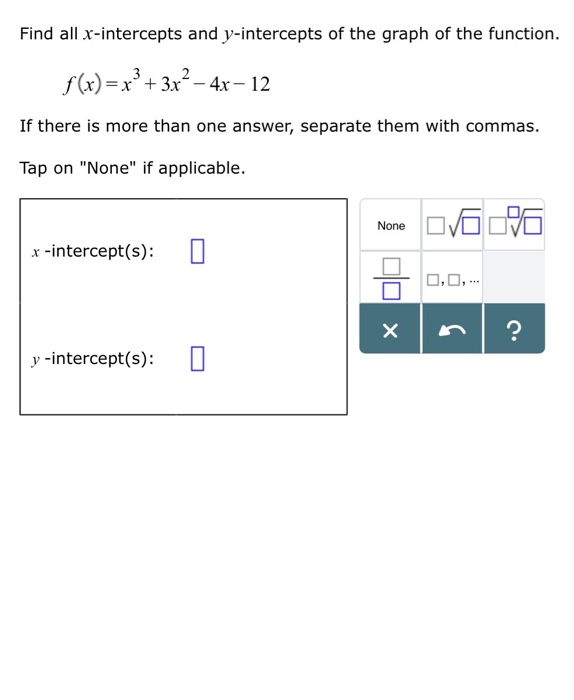



Find All X Intercepts And Y Intercepts Of The Graph Chegg Com
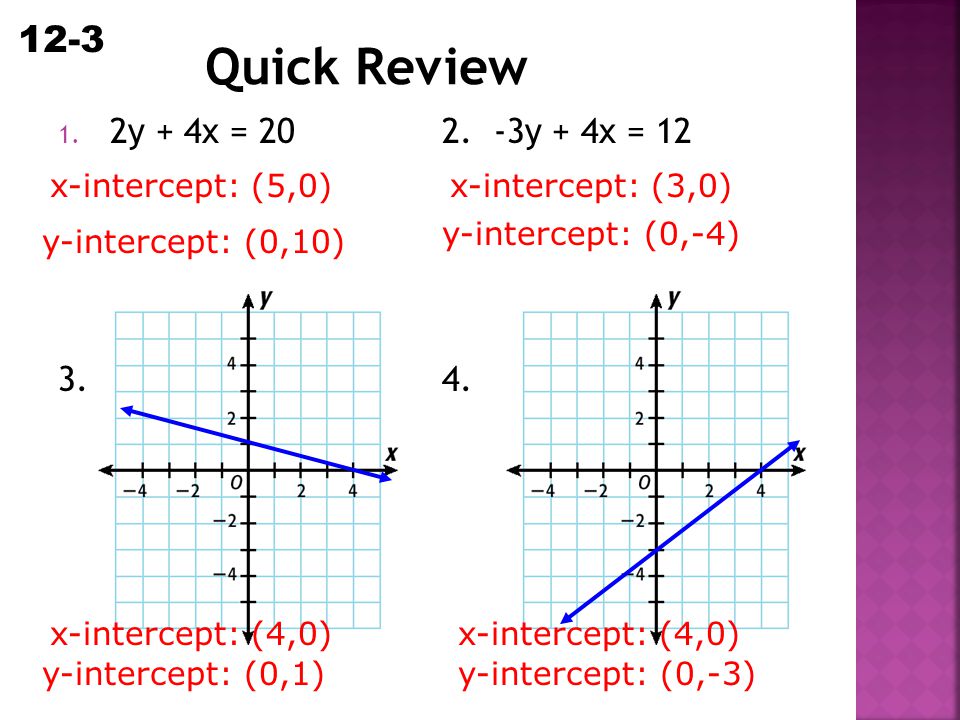



Learn To Use Slopes And Intercepts To Graph Linear Equations Ppt Video Online Download



Solution Y X2 4x 7 What Is The Y Intercept X Intercept Turning Point Please Write Down The Solution As Well Thanks




X Intercept Definition Overview Video Lesson Transcript Study Com



Tarantamath Licensed For Non Commercial Use Only Shanira 11



2




How To Sketch A Parabola Example 2 Y X 2 4x 12 Youtube
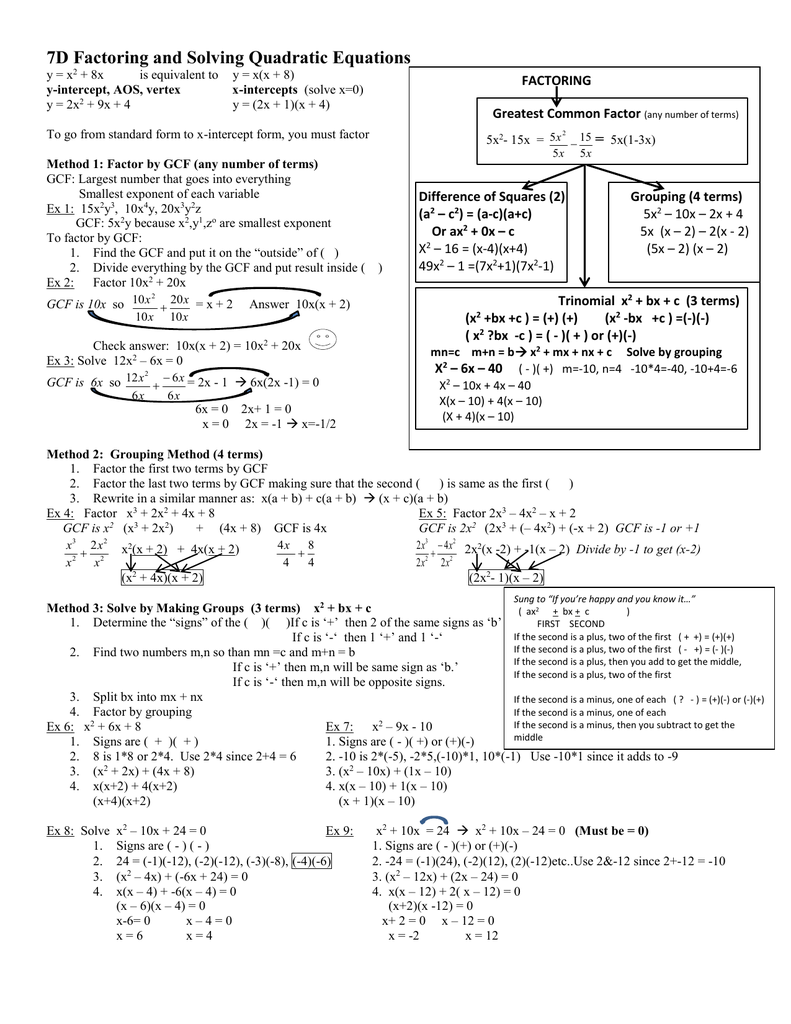



7d Factoring And Solving Quadratic Equations
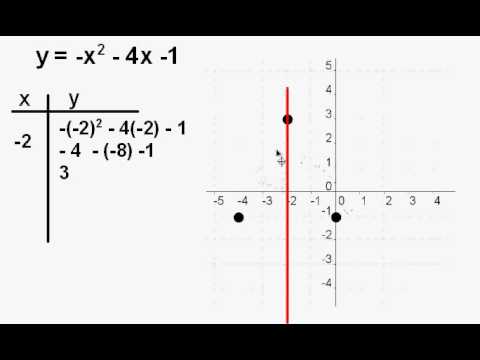



Graph The Parabola Y X 2 4x 1 Youtube



Norwalknhs Ss4 Sharpschool Com Userfiles Servers Server 763 File summer work Math Honors algebra 2 summer packet Pdf




How Many Intercepts Does The Graph Of Y 2x 2 5x 9 Brainly Com




Graph The Function F X X 2 4x 12 On The Coordinate Plane Do A B And C Explain Or Show How You Brainly Com
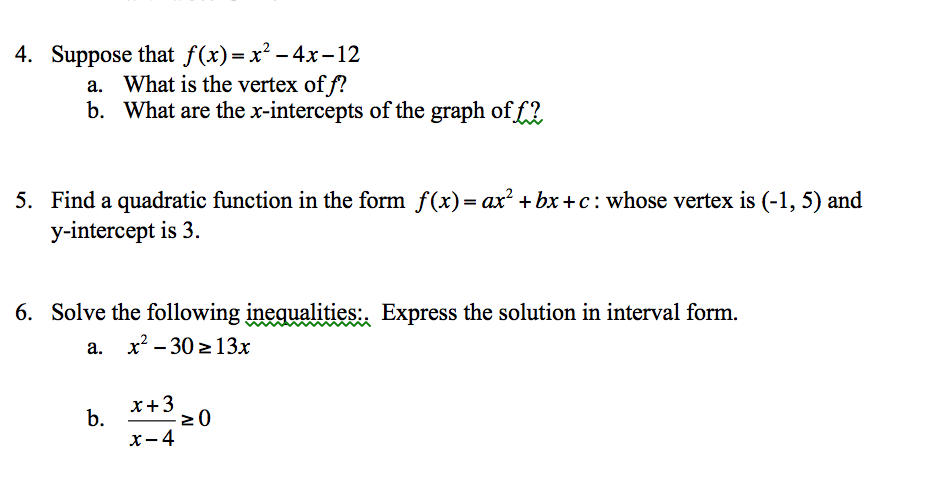



Suppose That F X X 2 4x 12 What Is The Vertex Chegg Com
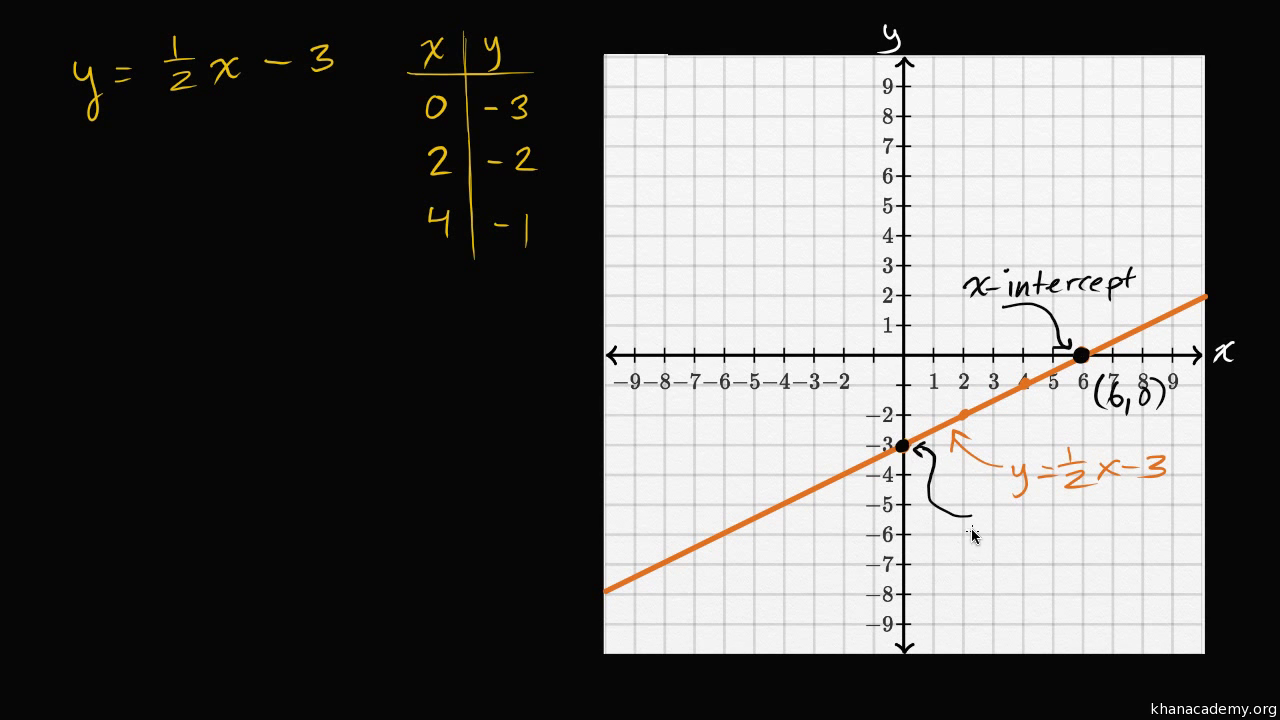



Intro To Intercepts Video Khan Academy
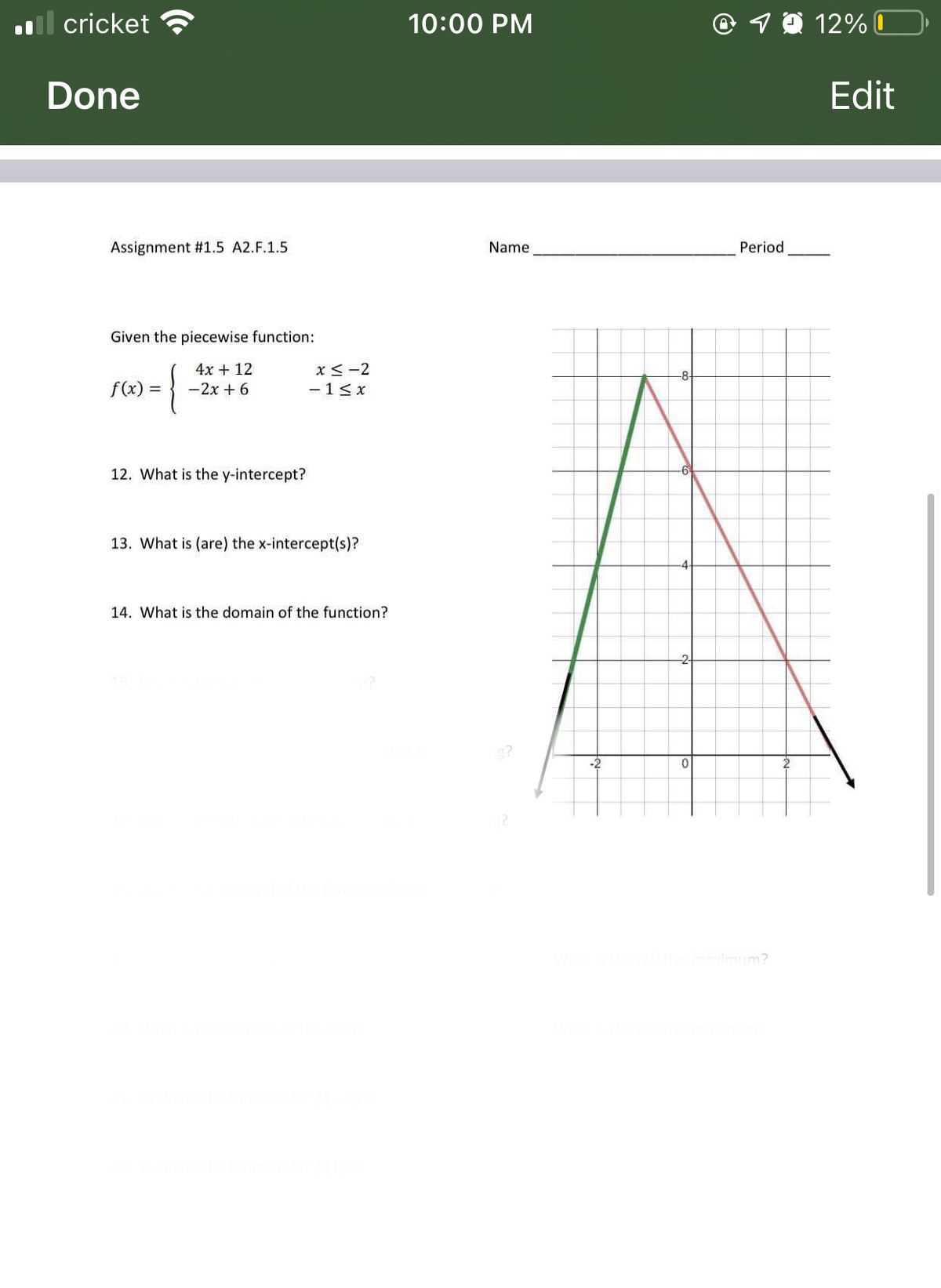



Answered Given The Piecewise Function 4x 12 Bartleby




Answered Consider The Quadratic Function F X Bartleby
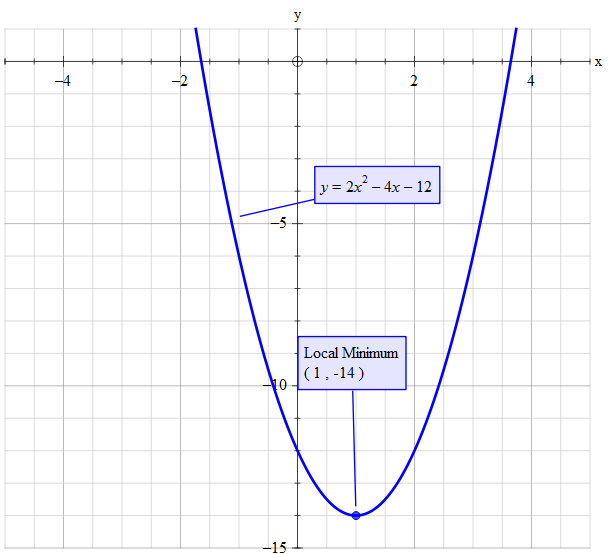



What Is The Vertex Of Y 2x 2 4x 12 Socratic
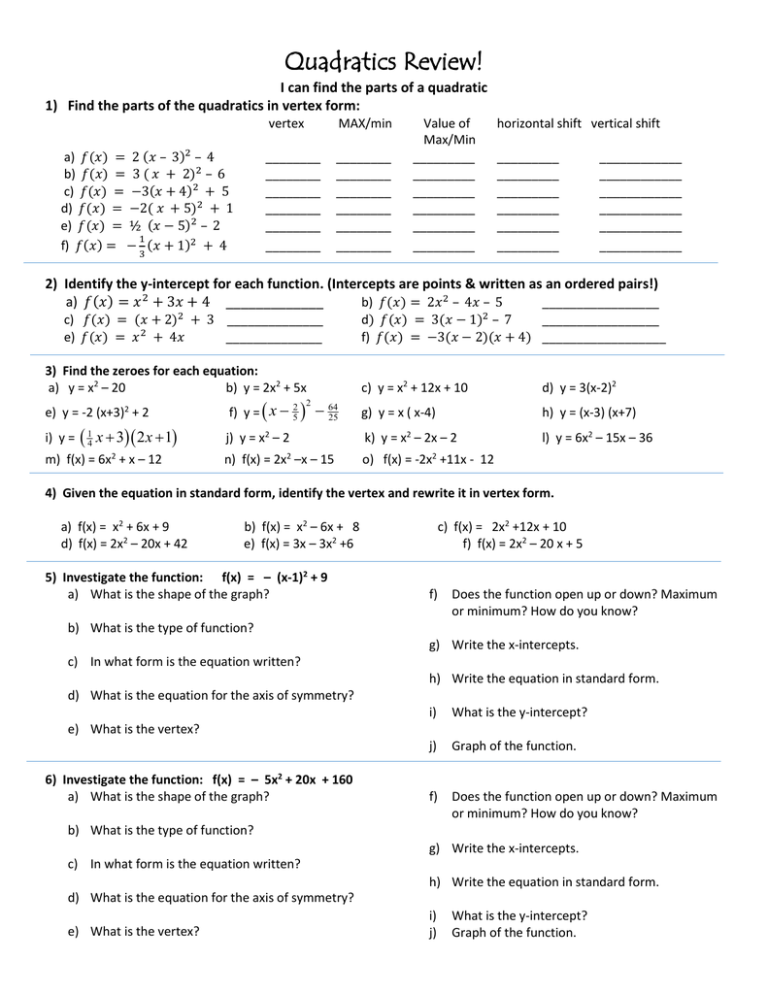



Quadratics Review 1 Quadrtics Test Review 1
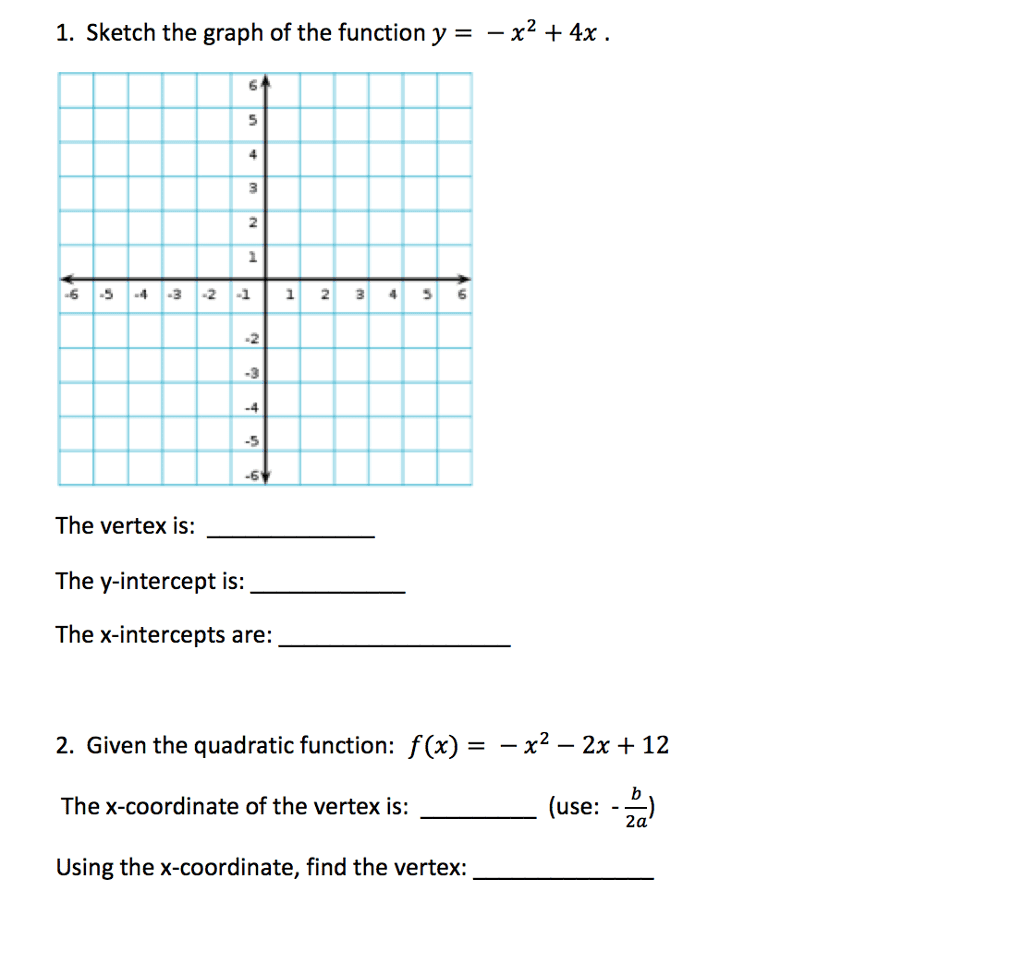



What S Your Question Pricing Log Insign Up Algebra 1 Answer 0 Watching 65 Views 10 Nov 19 Sketch The Graph Of The Function Y X 2 4x The Vertex Is The Y Intercept Is The X Intercepts Are Given The Quadratic Function F X X 2 2x 12 The X




X Intercepts Of Parabolas



1



What Are The X Intercept Y Intercept Vertical Asymptote And Horizontal Asymptote Of The Rational Function X 3 X 12 X 2 3x 4 Quora




W Steps A E To Graph F X X2 4x 12 0 72 2 Chegg Com




Consider The Quadratic Function F X X 2 4x 12 Determine The Following The Smallest Xx Intercept Is Brainly Com
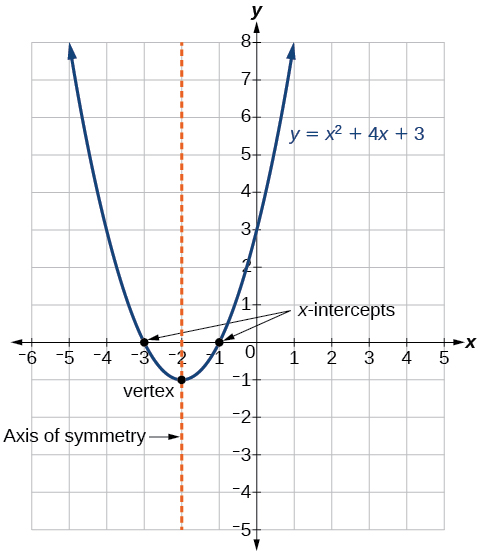



Quadratic Functions Algebra And Trigonometry




Plzz Help Graph The Function F X X 2 4x 12 On The Coordinate Plane A What Are The Brainly Com




What Are The Vertex And X Intercepts Of The Graph Of Y X2 4x 21 Select One Answer For The Vertex Brainly Com
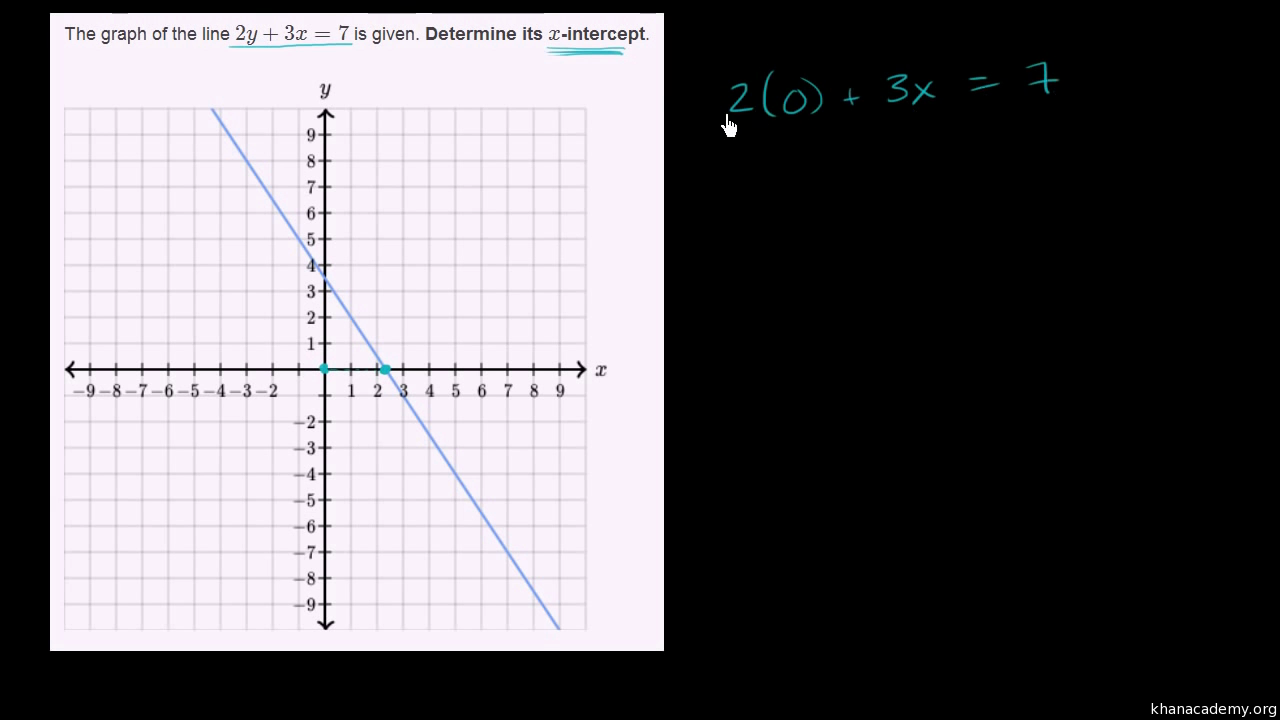



X Intercept Of A Line Video Khan Academy



Y X 2 4x 32 Axis Of Symmetry 2 Vertex 2 36 X Intercepts 4 0 8 0 How Dose The Axis Of Symmetry Relate To Enotes Com



Solution Suppose That F X X 2 4x 12 A What Is The Vertex Of F B What Are The X Intercepts Of The Graph Of F C Solve F X 12 For X What Points Are On The Graph




Answered 38 Find The Intercepts Of F X X Bartleby




Learn To Use Slopes And Intercepts To Graph Linear Equations Ppt Video Online Download




Standard Form X Intercepts Y Intercepts Ppt Download




What Are The X Intercepts Of The Graph Below Of The Function Below Y X 2 2x 15 Brainly Com
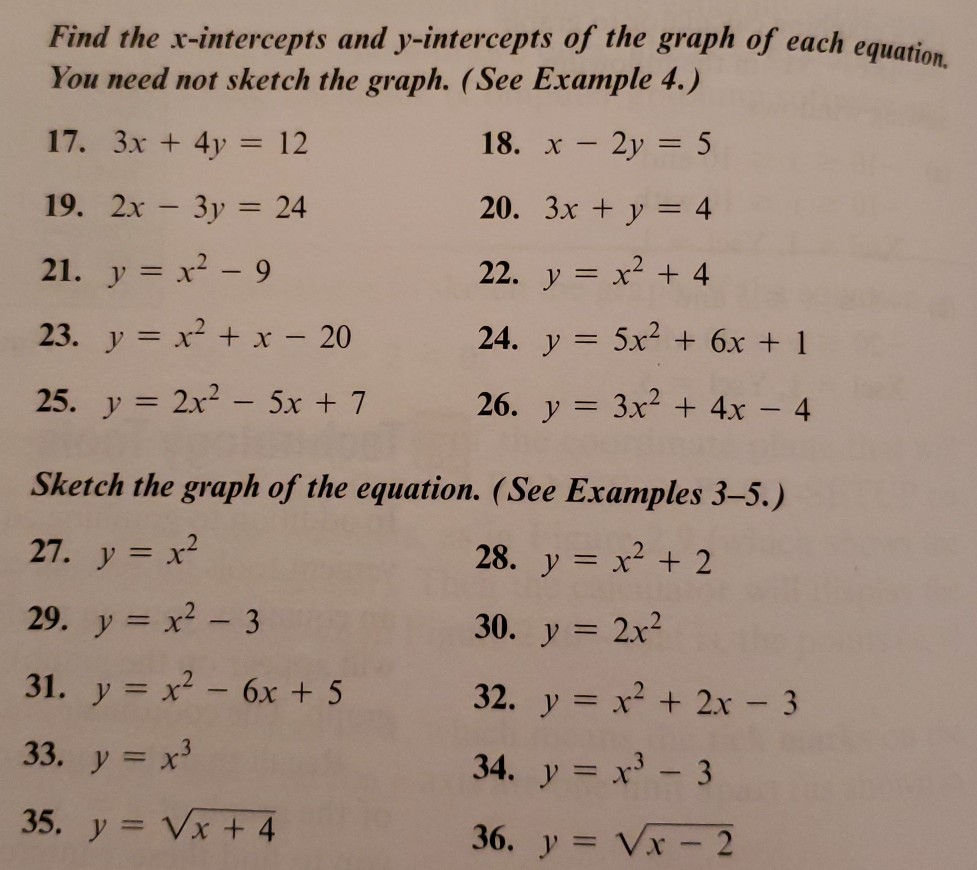



Find The X Intercepts And Y Intercepts Of The Graph Chegg Com
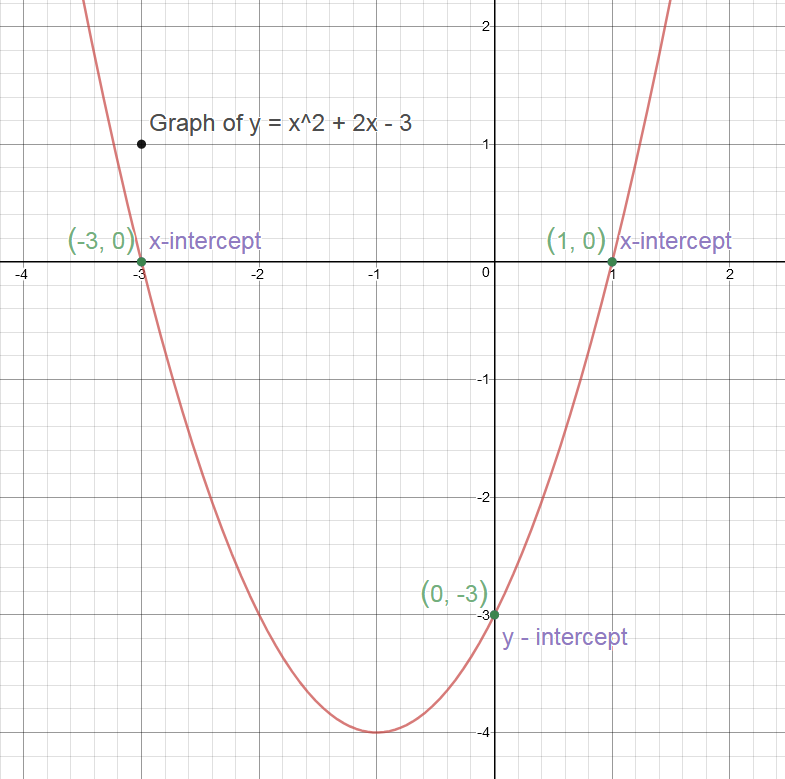



How Do You Find The X And Y Intercepts For Y X 2 2x 3 Socratic



Solve Quadratic Equations By Graphing Intercepts Expii
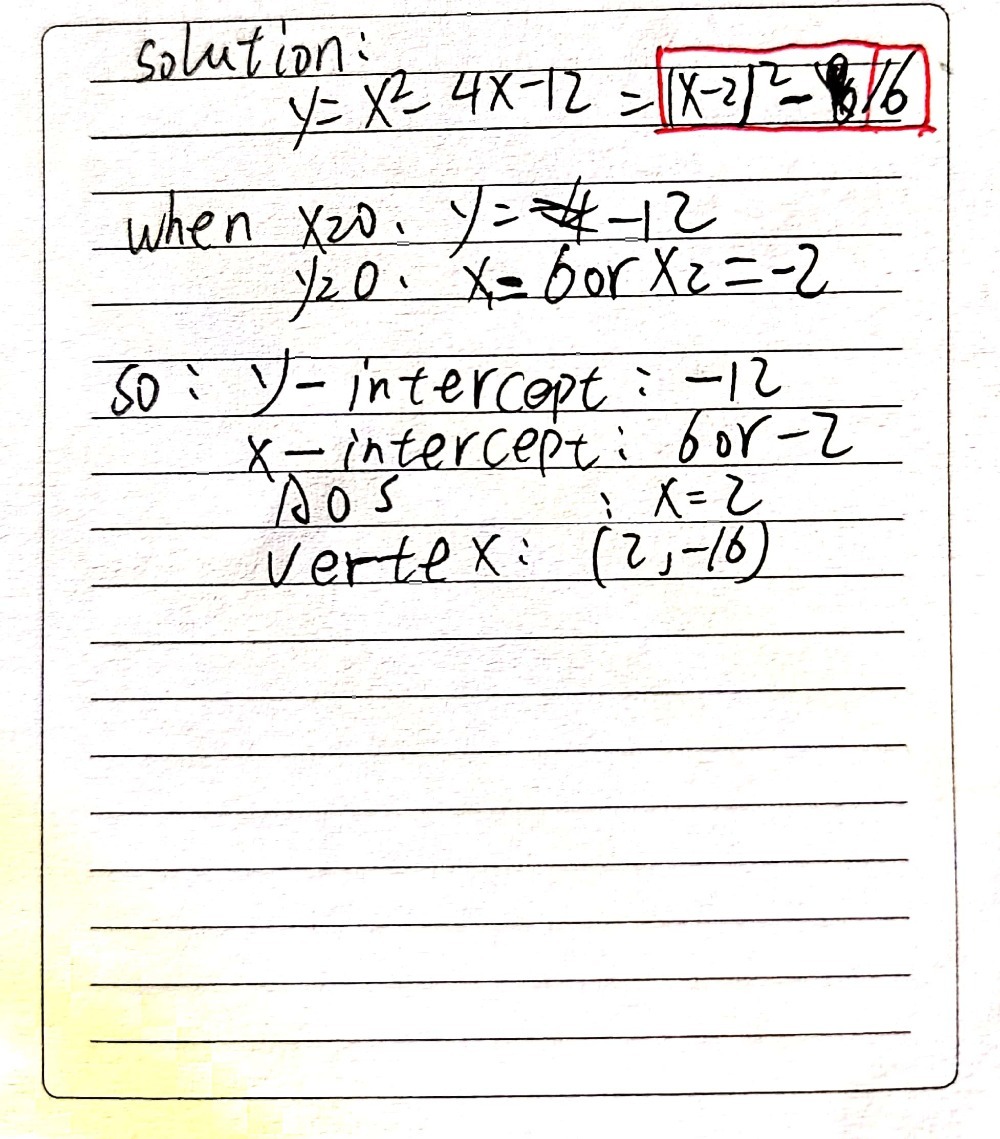



Find The Y Intercept X Intercepts Axis Of Symmet Gauthmath



Intro To Intercepts Video Khan Academy



Converting To Slope Intercept Form Video Khan Academy
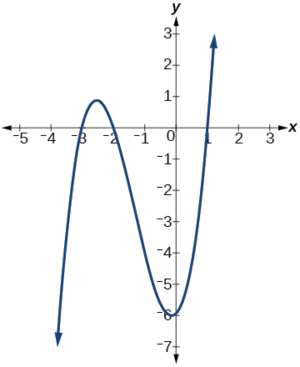



3 4 Graphs Of Polynomial Functions Mathematics Libretexts




From Yesterday In Case You Didn T Get It Ppt Download




For The Quadratic Function F X X2 4x 12 Chegg Com




Draw The Graph Of Linear Equation 4x 3y 12 At What Point The Graph Of The Equation Cut The X Youtube
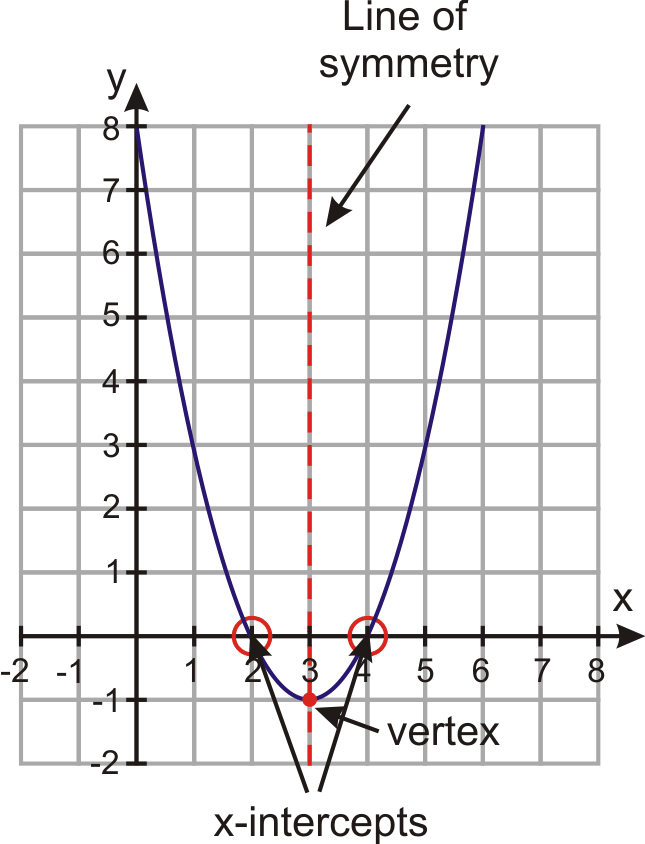



Graphs Of Quadratic Functions In Intercept Form Read Algebra Ck 12 Foundation




1 2 The Graphs Of Quadratic Equations




Graph Graph Equations And Equation Systems With Step By Step Math Problem Solver



Unique Quadratic Equation In The Form Y Ax 2 Bx C
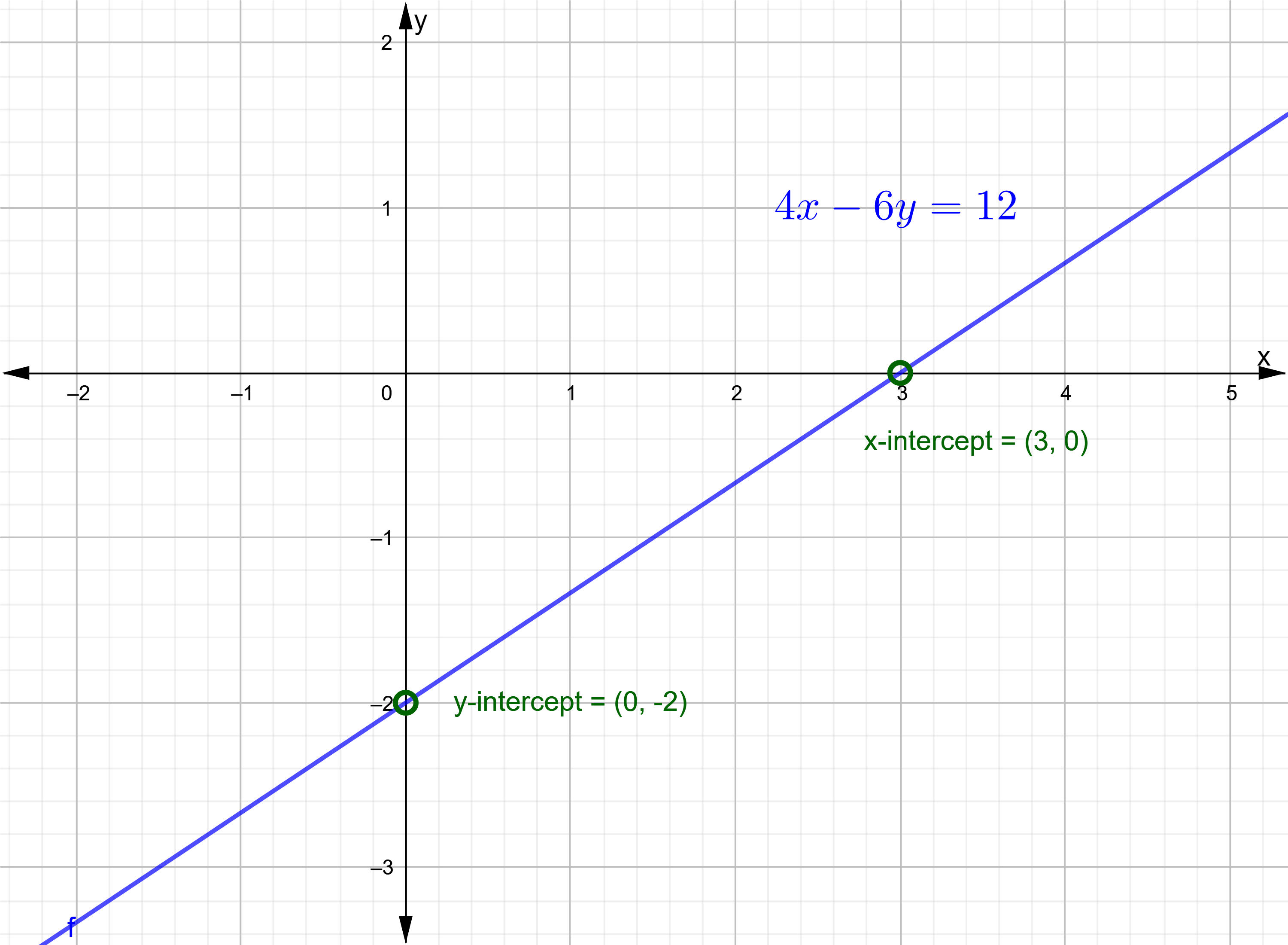



Consider The Equation 4x 6y 12 Explain Clearly How To Find The X And Y Intercepts Homework Help And Answers Slader
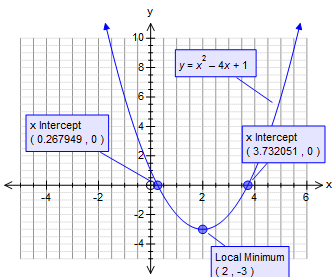



What Is The Vertex Of Y X 2 4x 1 Socratic
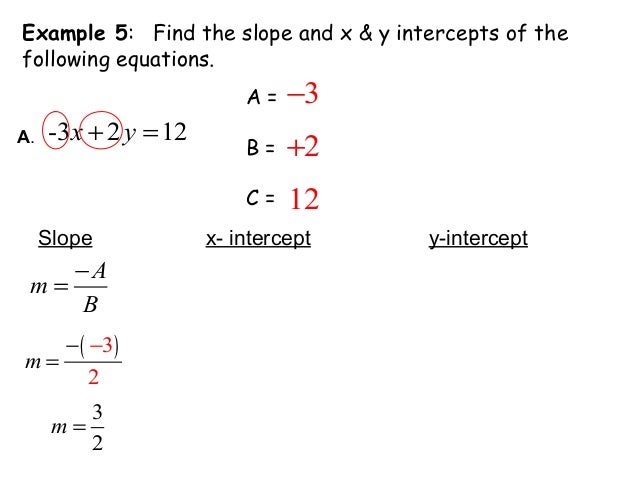



M8al Iif 2



Solved Question 1 Graph The Function F X X 2 4x 12 On The Coordinate Plane A What Are The X Intercepts And Y Intercept B What Is T Course Hero




3 For Y X2 4x 12 0 Find The Y Intercept Chegg Com




How Do You Graph Quadratic Functions Y X 2 4x 7 Socratic




Step To Draw Y X 2 4x 1and Find Solution To Quadratic Equation Y X 2 5x 4 Youtube
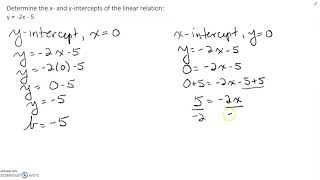



Mfm2p



0 件のコメント:
コメントを投稿