The focal length, the semilatus rectum is =,;Divide y8 by 2 x=\frac {\sqrt {2y16}} {2} x=\frac {\sqrt {2y16}} {2} Take the square root of both sides of the equation 2x^ {2}8=y Swap sides so that all variable terms are on the left hand side 2x^ {2}8y=0 Subtract y from both sides 2x^ {2}y8=0 Quadratic equations like this one, with an x^ {2} term but no x term, can still be A conducting wire bent in the form of a parabola y^2=2x , carries a current I=2A as shown in figure The wire is subjected to a uniform magnetic field vecB=2hatk tesla

Parabola Parent Function Mathbitsnotebook A1 Ccss Math
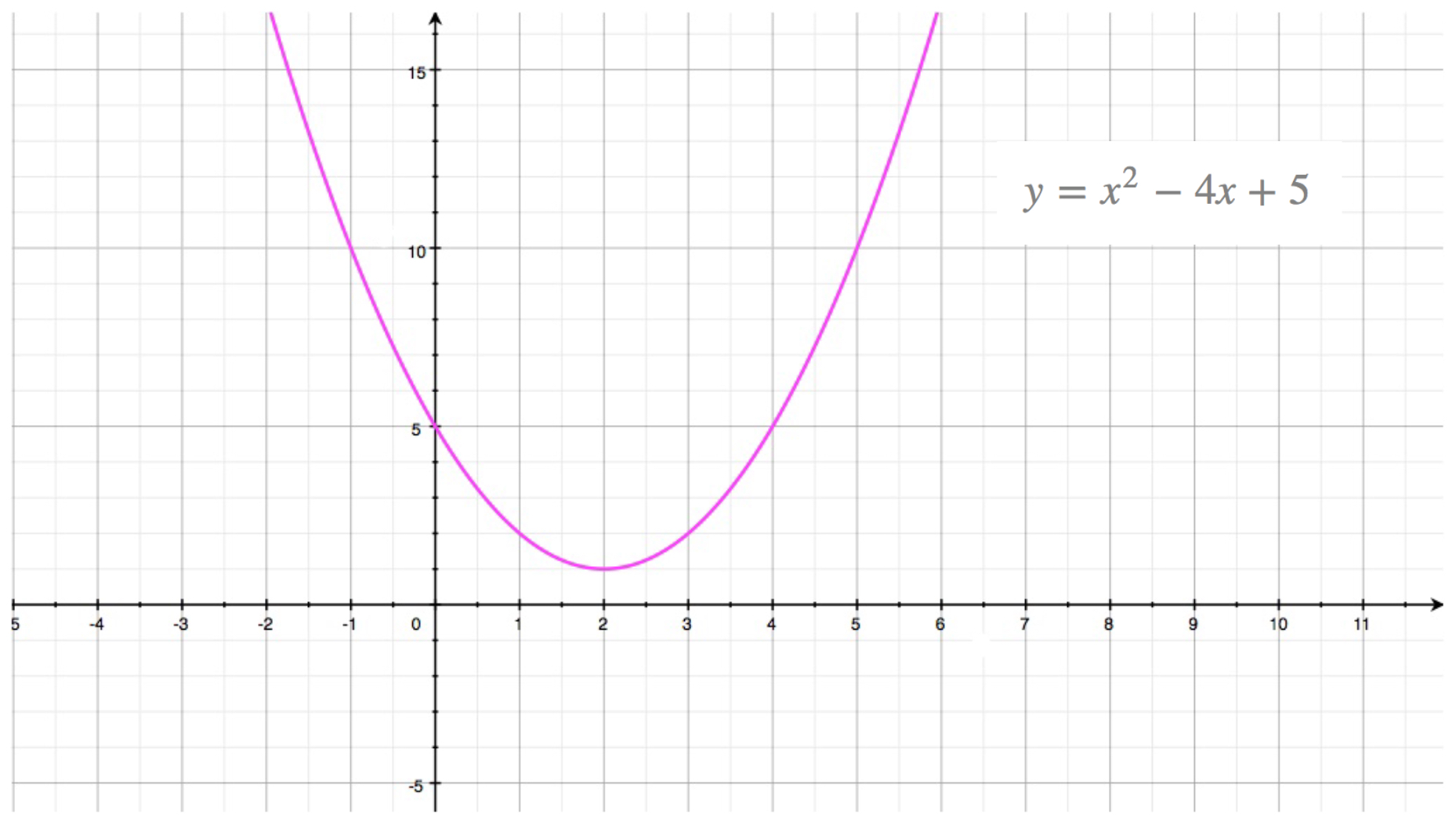
Y=x^2-2x-8 parabola
Y=x^2-2x-8 parabola-Parabola, Finding the Vertex 41 Find the Vertex of y = 2x 22x5 Parabolas have a highest or a lowest point called the Vertex Our parabola opens down and accordingly has a highest point (AKA absolute maximum) We know this even before plotting "y" because the coefficient of the first term, 2 , is negative (smaller than zero)The vertex is (,),




Graphing Parabolas
{eq}y= 2x^2 4x 8 = 0 {/eq} Parabolas The equation of a parabola is a quadratic equation whose points are all the same distance from a focus point and a directrix lineAlso graph the parabola If you can please include the points that I have to graph/plot that would be great I know how to get the vertex but I always mess up when it comes to graphing the exact point or points Answer by ewatrrr() (Show Source)This problem has been solved!
Exploration of Parabolas By Thuy Nguyen In this exploration we want to see what happens when we construct the graphs for the parabola y = ax 2 bx c with different values of a, b, and c We'll start first by constructing the graphs for y = ax 2 with different values of a The following are graphs for a = 2, 1, 2, in blue, purple, and red respectivelySubtract y from both sides 2x^ {2}8x1y=0 2 x 2 8 x 1 − y = 0 This equation is in standard form ax^ {2}bxc=0 Substitute 2 for a, 8 for b, and 1y for c in the quadratic formula, \frac {b±\sqrt {b^ {2}4ac}} {2a} This equation is in standard form a x 2 b x c = 0First we solve the 2 equations to find where the parabola and line intersect y^2= 2x=y On solving we get y=0,x=0 and y=1,x=1/2 The picture is like this The shaded region is now the required area So by using integral calculus of areas Integrate √ (2x) 2x putting limits 0 to 05
Where the line for the equation y = 2x 2 intersects the parabola Answers 1 Get Other questions on the subject Mathematics Mathematics, 1530, morganwendel126 Mary used 1/7 of a spool of ribbon to complete 4 projects she used the same amount of ribbon on each project how much ribbon did she use on each project? The equation of the parabola #y=x^2# shifted 5 units to the right of equation, what is the new How do you sketch the graph of #y=3x^2# and describe the transformation?Nathalien5193 está esperando tu ayuda Añade tu respuesta y gana puntos Nuevas preguntas de Matemáticas AYUDA!




Graphing Parabolas



Solution Graph Y 2x 2 4x
Find stepbystep Calculus solutions and your answer to the following textbook question Does the parabola y = 2x^2 13x 5 have a tangent line whose slope is 1?Y=2x^2 What is the vertex? The equation of the parabola whose focus is (1, 1) and the directrix is x y 7 = 0 is A x^2 y^2 2xy 18x 10y = 0 asked Jul 19 in Parabola by Maanas ( 259k points) parabola




How To Draw Y 2 X 2



Solution The Equation Of The Parabola C Is Y 2x 2 5x The Equation Of The Line L Is Y 4x 1 0 Sketch C And L On The Same Diagram
Free Parabola calculator Calculate parabola foci, vertices, axis and directrix stepbystep parabolaequationcalculator y=2x^{2} en Related Symbolab blog posts Practice Makes Perfect Learning math takes practice, lots of practice Just like running, itIf so, find an equation for the line and the point of tangency If not, why not?Si la parábola y=2x^2(b)x9 tien su vértice en el eje de las abscisas ¿cuál es el valor de b?




Focus Of Parabola Y 2x 2 X



Solution Given Y 2x 2 4x Thanks A Find The Y Intercept B Find The X Intercepts If Any C Find The Vertex D Sketch The Parabola
Short demo on graphing a parabola by finding the vertex and yintercept, and using the axis of symmetryI have an equation right here it's a second degree equation it's a quadratic and I know it's graph is going to be a parabola this was a review that means it looks something like this or it looks something like that because the coefficient on the x squared term here is positive and it's going to be an upwardopening parabola and I am curious about the vertex of this parabola and if I haveThe parabola `y=x^(2)pxq` intersects the straight line `y=2x3` at a point with abscissa 1 If the distance between the vertex of the parabola nad the Xax




Graphing Parabolas




How To Draw Y 2 X 2
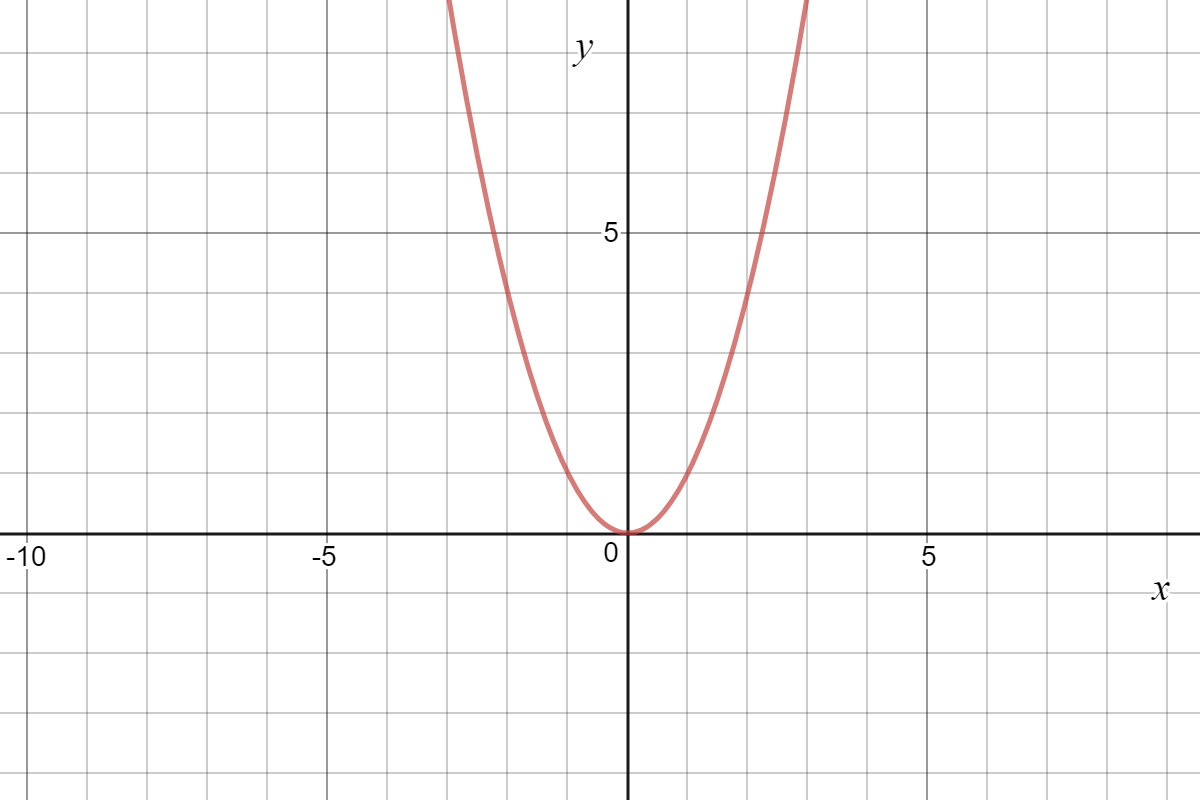
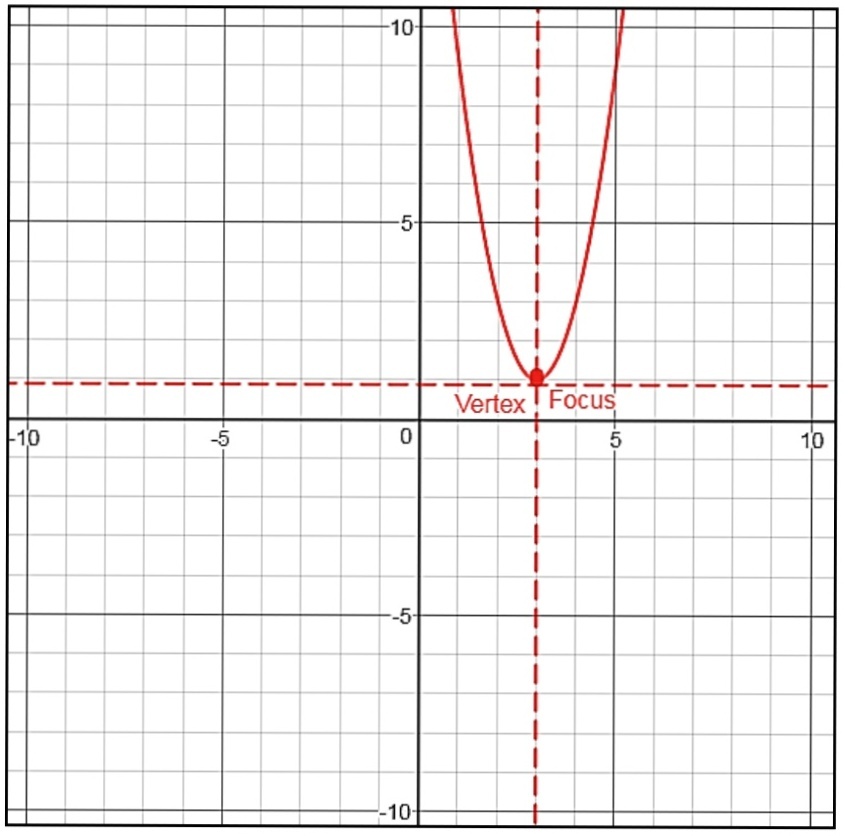
Free Parabola Vertex calculator Calculate parabola vertex given equation stepbystep This website uses cookies to ensure you get the best experienceGraph y=2x^2 Find the properties of the given parabola Tap for more steps Rewrite the equation in vertex form Tap for more steps Complete the square for The directrix of a parabola is the horizontal line found by subtracting from the ycoordinate of the vertex if the parabola opens up or downThe focus of a parabola can be found by adding to the ycoordinate if the parabola opens up or down Substitute the known values of , , and into the formula and simplify Find the axis of symmetry by finding the line that passes through the vertex and the focus




Quadratic Function



Solution Graph The Quadratic Equation Y 2x 2 What Is The Vertex Also Graph The Parabola If You Can Please Include The Points That I Have To Graph Plot That Would Be G
The first thing I recognize in that equation is the y 2 term, which tells me it will be a parabola (It won't be a circle, ellipse or hyperbola because there is an x term, but no x 2 term See Conic Sections) Let's start with the most basic parabola y = x 2 and build up to the required answer Example 1 y = x 2The directrix of a parabola is the vertical line found by subtracting from the xcoordinate of the vertex if the parabola opens left or right Substitute the known values of and into the formula and simplify Use the properties of the parabola to analyze and graph the parabolaEvery parabola has an axis of symmetry which is the line that divides the graph into two perfect halves On this page, we will practice drawing the axis on a graph, learning the formula, stating the equation of the axis of symmetry when we know the parabola's equation



Graphs Of Functions Y X2 Y 2x2 And Y 2x2 In Purple Red And Blue Respectively



Exploration Of Parabolas
Precalculus Find the Vertex y=2x^2 y = 2x2 y = 2 x 2 Rewrite the equation in vertex form Tap for more steps Complete the square for 2 x 2 2 x 2 Tap for more steps Use the form a x 2 b x c a x 2 b x c, to find the values of a a, b b, and c c a = 2, b = 0, c = 0 a = 2, b = 0, c = 0Parabola Calculator This calculator will find either the equation of the parabola from the given parameters or the axis of symmetry, eccentricity, latus rectum, length of the latus rectum, focus, vertex, directrix, focal parameter, xintercepts, yintercepts of the entered parabola To graph a parabola, visit the parabola grapher (choose theThe "formula" for a parabola (in the conic sections form) is mathx^2=4cy/math (although some textbooks use mathp/math instead of mathc/math, so mathx^2=4py/math) If you put your equation into that form you can make some observatio
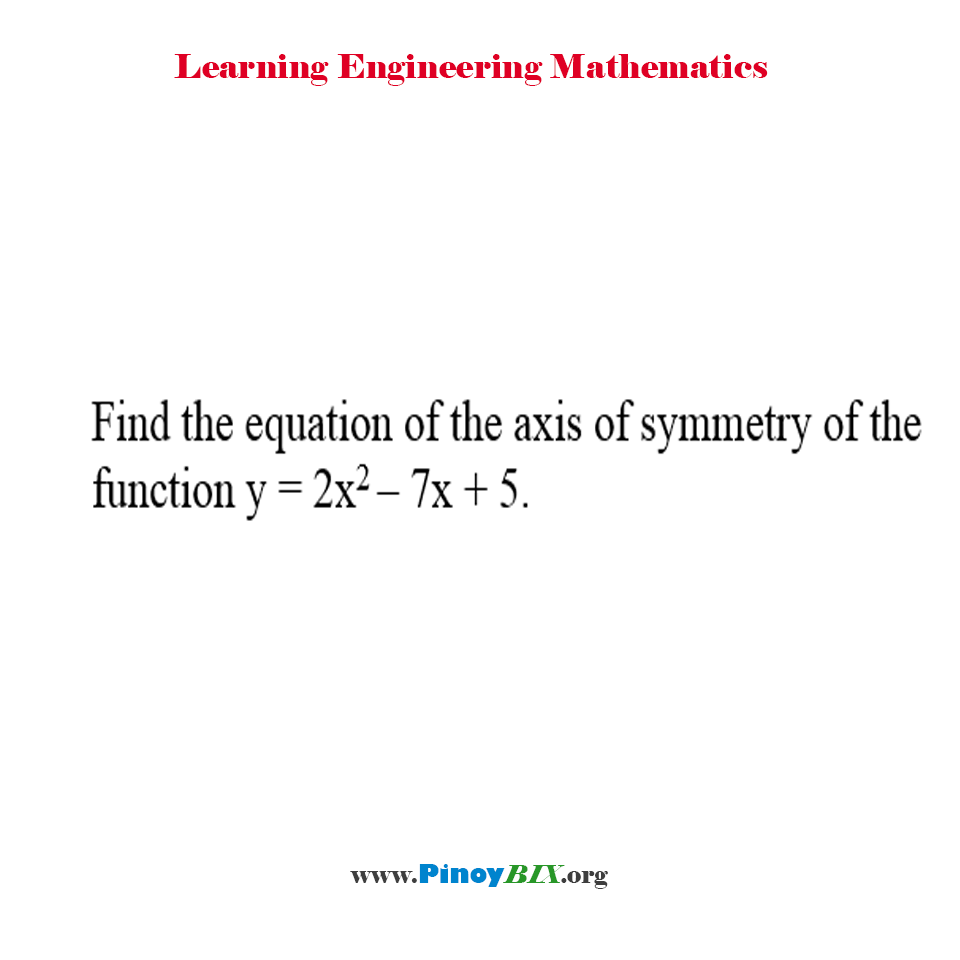



Solution Find The Equation Of The Axis Of Symmetry Of The Function Y 2x 2 7x 5




Consider The Parabola Whose Equation Is Y X 2 4x And The Line Y 2x B Then Whichfollowing Is Are Correct
Free Parabola calculator Calculate parabola foci, vertices, axis and directrix stepbystep This website uses cookies to ensure you get the best experience parabolaequationcalculator y=2x^{2}8x5 en Related Symbolab blog posts Practice, practice, practiceGraph y=2x^212x10 y = −2x2 12x − 10 y = 2 x 2 12 x 10 Find the properties of the given parabola Tap for more steps Rewrite the equation in vertex form Tap for more steps Complete the square for − 2 x 2 12 x − 10 2 x 2 12 x 10 Tap for more steps Use the form a x 2 b x c a x 2 b x c, to find theLet's graph y=2x 2x (red) and y=2x 24x (blue) The vertex when a =2 and b =1 is at x=¼, and the vertex when a =2 and b =4 is x=1 Now, we see that our hypothesis is not completely correct, for – b /2 for the first graph would give us ½, but we have ¼



Untitled Document




Graph The Parabola And Give Its Vertex Axis X Intercepts And Y Intercept Y 2x 2 8x 16 Study Com
What happens when we graph y=2x 2 b x for different values of b?See all questions in Vertical Shifts of Quadratic FunctionsReleased under CC BYNCSA http//creativecommonsorg/licenses/byncsa/30/legalcodeGraphing a basic parabola using y=2x^22 to introduce yk Part 2 of a 4



Sketching Quadratics



Graph Y 2x 2 3x 5 Math Homework Answers
The parabola has the equation y=2x^2x If y=ax^2bx then y'=2axb This gives us our slope of y at any given x So at the point (1,1), the slope must be y'=2a(1)b=2ab We know the slope must also be 3 at the point (1,1), to match the linear equation given Thus, these two slope values must be equal 2ab=3 1 We also know that (1,1) is a point on the parabola, so it mustParabola, Finding the Vertex 31 Find the Vertex of y = 2x 2 x7 Parabolas have a highest or a lowest point called the Vertex Our parabola opens down and accordingly has a highest point (AKA absolute maximum) We know this even before plotting "y" because the coefficient of the first term, 2 , is negative (smaller than zero)Let y=x1 is axis of parabola, yx4=0 is tangent of same parabola at its vertex and y=2x3 is one of its tangents Then find the focus of the parabola
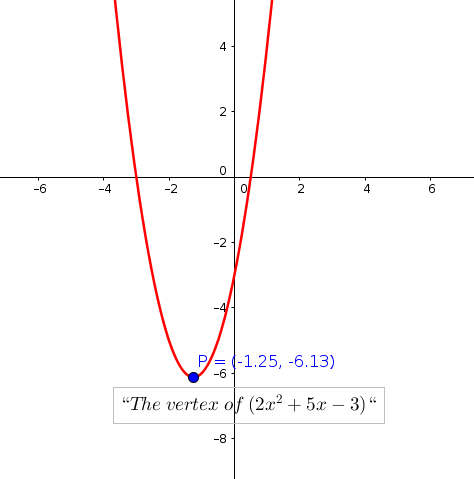



What Is The Vertex Form Of Y 2x 2 5x 3 Socratic




Parabola Parent Function Mathbitsnotebook A1 Ccss Math
Question 5 Find the minimum distance between the line y = 2x 2 and the parabola x =y?Expresa el número entero 16 de tal manera que a) 16 sea el producto de dos números enteros (Considera todos los casos posibles)Parabola, Finding the Vertex 41 Find the Vertex of y = 2x 2 x3 Parabolas have a highest or a lowest point called the Vertex Our parabola opens up and accordingly has a lowest point (AKA absolute minimum) We know this even before plotting "y" because the coefficient of the first term, 2 , is positive (greater than zero)



Parabolas



2
where (h,k) are the coordinates of the vertex and a is a multiplier to obtain this form use the method of completing the square ∙ the coefficient of the x2 term must be 1 ⇒ y = − 2(x2 − 6x 13 2) ∙ add/subtract (1 2 coefficient of the xterm)2 to x2 −6xSi el vídeo te sirvió comparte, comenta y suscríbete eso nos ayudaría mucho para seguir creciendo y ofrecer materiales de estudio de mejor calidad RecordarParabola, Finding the Vertex 31 Find the Vertex of y = 2x 25x5 Parabolas have a highest or a lowest point called the Vertex Our parabola opens up and accordingly has a lowest point (AKA absolute minimum) We know this even before plotting "y" because the coefficient of the first term, 2 , is positive (greater than zero)




Parabola Y X 2 Geogebra




Find The Vertex Of The Parabola Y 2x2 10x 8 Brainly Com
The Vertex of a Parabola There are two methods to obtain the coordinates of the vertex of a parabola In the first method, we can use the direct result x= −( b 2a) x = − ( b 2 a) whileSee the answer See the answer See the answer done loading Show transcribed image text Forget 0 that's a local maximum So, the distance from the parabola to C is ‖(1 2, 1) − (3 2, 0)‖ = √2 Any point of the parabola is of the type (x, Y) with y2 = 2x The distance between (x, y) and (15, 0) is √(x − 15)2 y2 = (x − 15)2 2x You have to find the minimum value of this quantity over all x ≥ 0 x = (y2 / 2
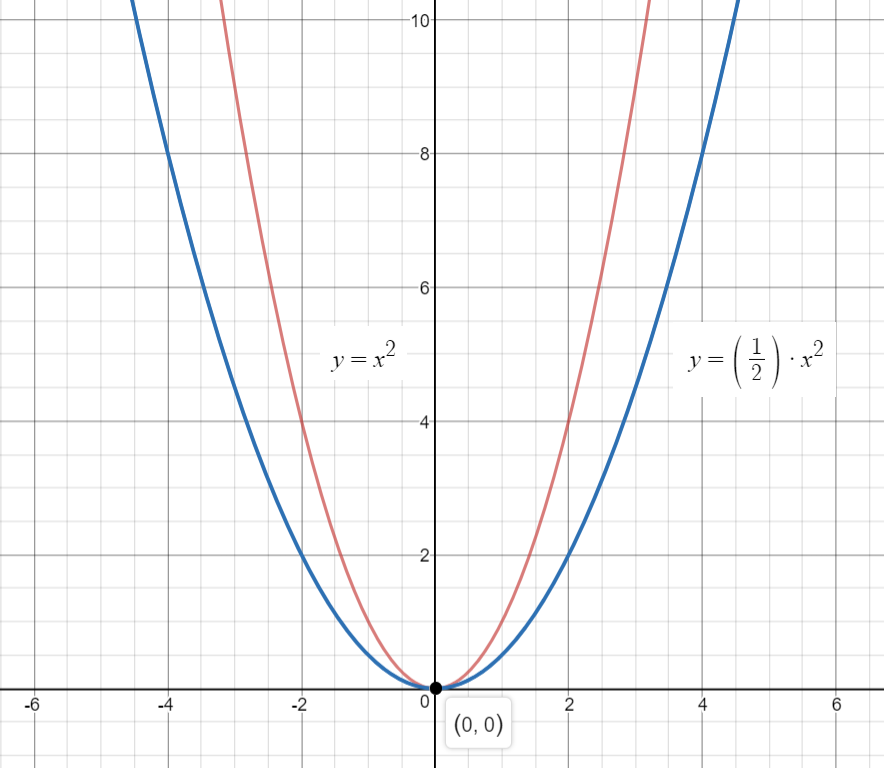



How Do You Sketch The Graph Of Y 1 2x 2 And Describe The Transformation Socratic



Draw The Graph Of Y 2x 2 X 6 Mathskey Com
Refer explanation section Given y=2x^24 To find the vertex, rewrite the function as y=2x^x4 xcoordinate of the vertex x=(b)/(2a)=0/(2 xx 2)=0 y coordinate of the vertex At x=0; Let's say you have the following equation y = 2x 21 This parabola will be shaped like a "U" because the a value (2) is positive If the equation has a squared y term instead of a squared x term, the parabola will be oriented horizontally and open sideways, to the right or left, like a "C" or a backward "C"Parabola, Finding the Vertex 31 Find the Vertex of y = 2x 212x5 Parabolas have a highest or a lowest point called the Vertex Our parabola opens up and accordingly has a lowest point (AKA absolute minimum) We know this even before plotting "y" because the coefficient of the first term, 2 , is positive (greater than zero)




How Do You Find The Vertex Of The Parabola Y 2x 2 8x 7 Socratic



Mathscene Functions 1 Lesson 3
Calculadora gratuita para parábolas Calcular los focos de una parábola, sus vértices, ejes y su directriz paso por pasoThe previous section shows that any parabola with the origin as vertex and the y axis as axis of symmetry can be considered as the graph of a function =For > the parabolas are opening to the top, and for < are opening to the bottom (see picture) From the section above one obtains The focus is (,),;This video shows how to take the graph of y = x^2 and stretch it vertically by a factor of 2




Graph The Quadratic Functions Y 2x 2 And Y 2x 2 4 On A Separate Piece Of Paper Using Those Graphs Compare And Contrast The Shape And Position Of The Graphs Study Com
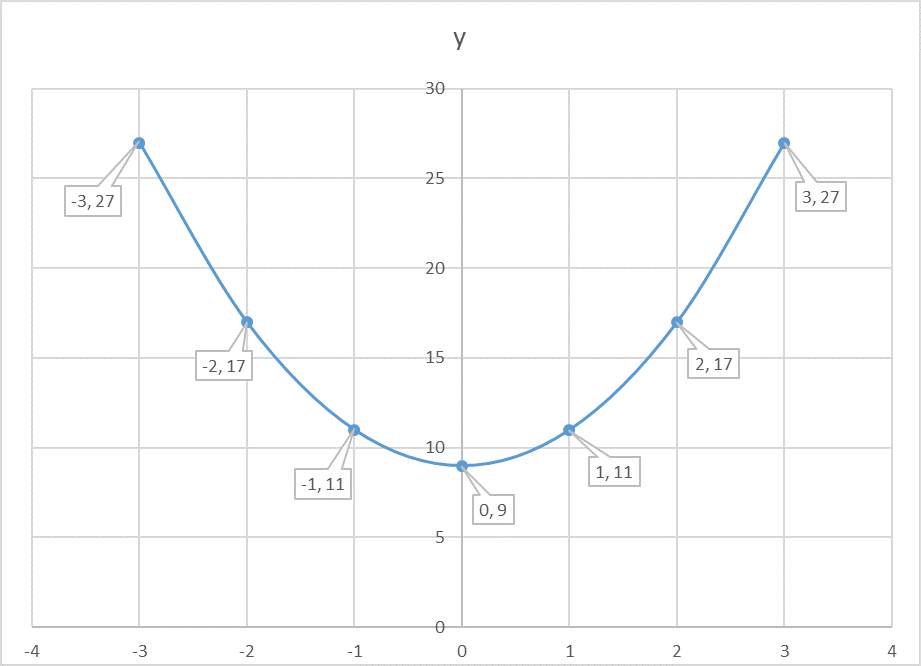



How Do You Graph The Parabola Y 2x 2 9 Socratic
Click here👆to get an answer to your question ️ The focus of the parabola y = 2x^2 x is Join / Login maths The focus of the parabola y = 2 x 2 x is A If A (2, 0) is the vertex and the yaxis is the directrix of a parabola, then its focus is View solutionSubtract y from both sides 2x^ {2}4xy=0 2 x 2 − 4 x − y = 0 This equation is in standard form ax^ {2}bxc=0 Substitute 2 for a, 4 for b, and y for c in the quadratic formula, \frac {b±\sqrt {b^ {2}4ac}} {2a} This equation is in standard form a x 2 b x c = 0Y=2(0)^(0)4=4 Vertex (0, 4) y Intercept (0, 4) To find the xintercept put y=0 2x^24=0 2x^2=4 x^2=(4)/2 x=sqrt(4)/2 The function has imaginary roots It means, it doesn't have x



Graphing And Properties Of Parabolas Kuta Software




Draw The Graph Of Y 2x 2 And Hence Solve 2x 2 X 6 0
Find the minimum distance between the line y = 2x 2 and the parabola x =y?




How Do You Find The Vertex Of Y 2x 2 4x Socratic



Mathscene Functions 1 Lesson 3




Solution Find The Equation Of The Axis Of Symmetry Of The Function Y 2x 2
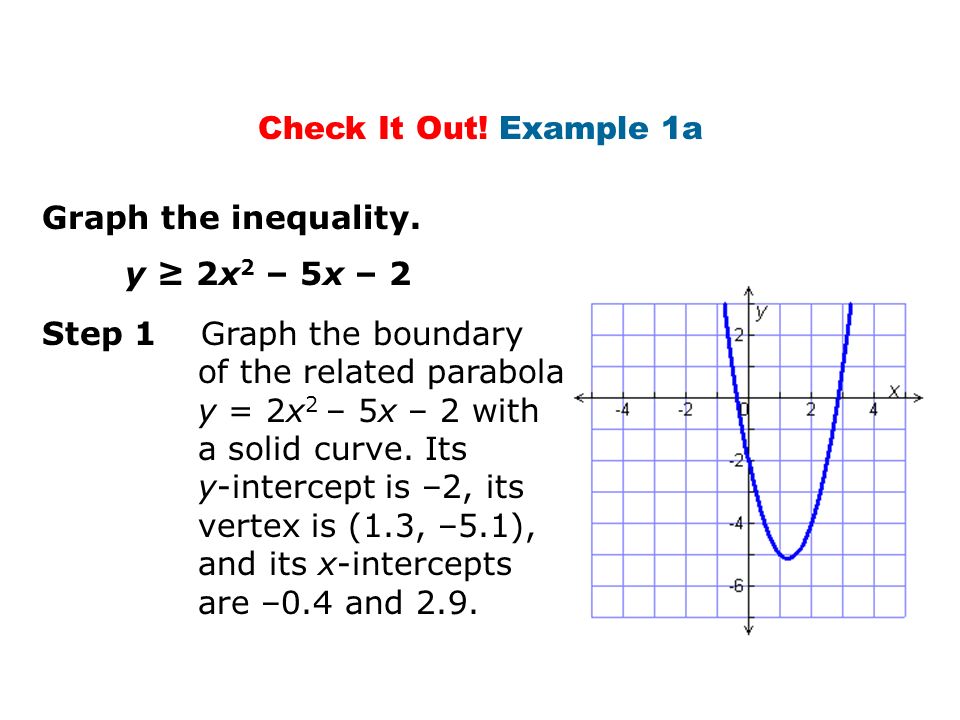



Warm Up 1 Graph The Inequality Y 2x 1 Solve Using Any Method 2 X 2 16x 63 X 2 8x 3 7 Ppt Download
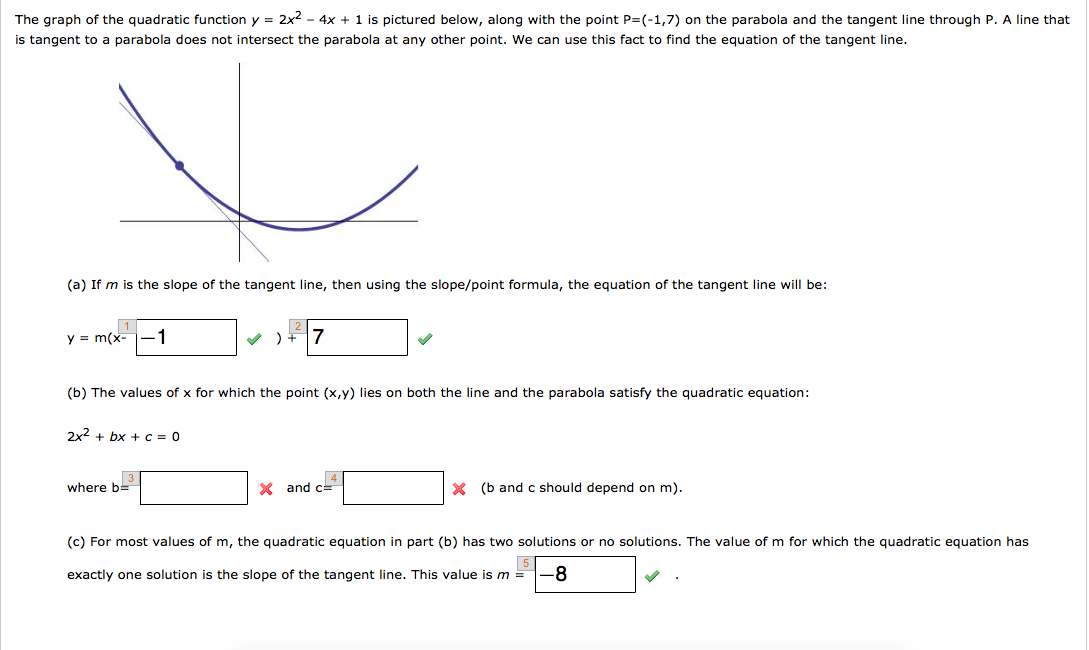



The Graph Of The Quadratic Function Y 2x 2 4x 1 Chegg Com




Graphing Parabolas



Parabolas And Cubics



Graph The Parabola 12 Y 2x2 5 A B 10 Chegg Com




Find The Vertex Of The Parabola Y 2x 2 12x 13 Blurtit



How Do You Graph Y 2x 2 4x 3 Mathskey Com



What S The Axis Of Symetry Vertex And Graph Y 2x 2 6x 1 Math Homework Answers




Quadratic Function



1



Quadratic Graph Example Y Ax C Expii




Mfg Graphing Parabolas




How To Graph A Parabola 13 Steps With Pictures Wikihow



For The Equation Y 2x 2 How Do I Find The Focus And The Directrix Of That Parabola Explaining All The Steps Quora



Graphs Of Functions Y X2 Y 2x2 And Y 2x2 In Purple Red And Blue Respectively




Parabolas




Please Help Thank You The Graph Of The Function Y X2 Is Shown How Will The Graph Change If Brainly Com




Graph The Parabola Y 2x 2 4x 1 Youtube
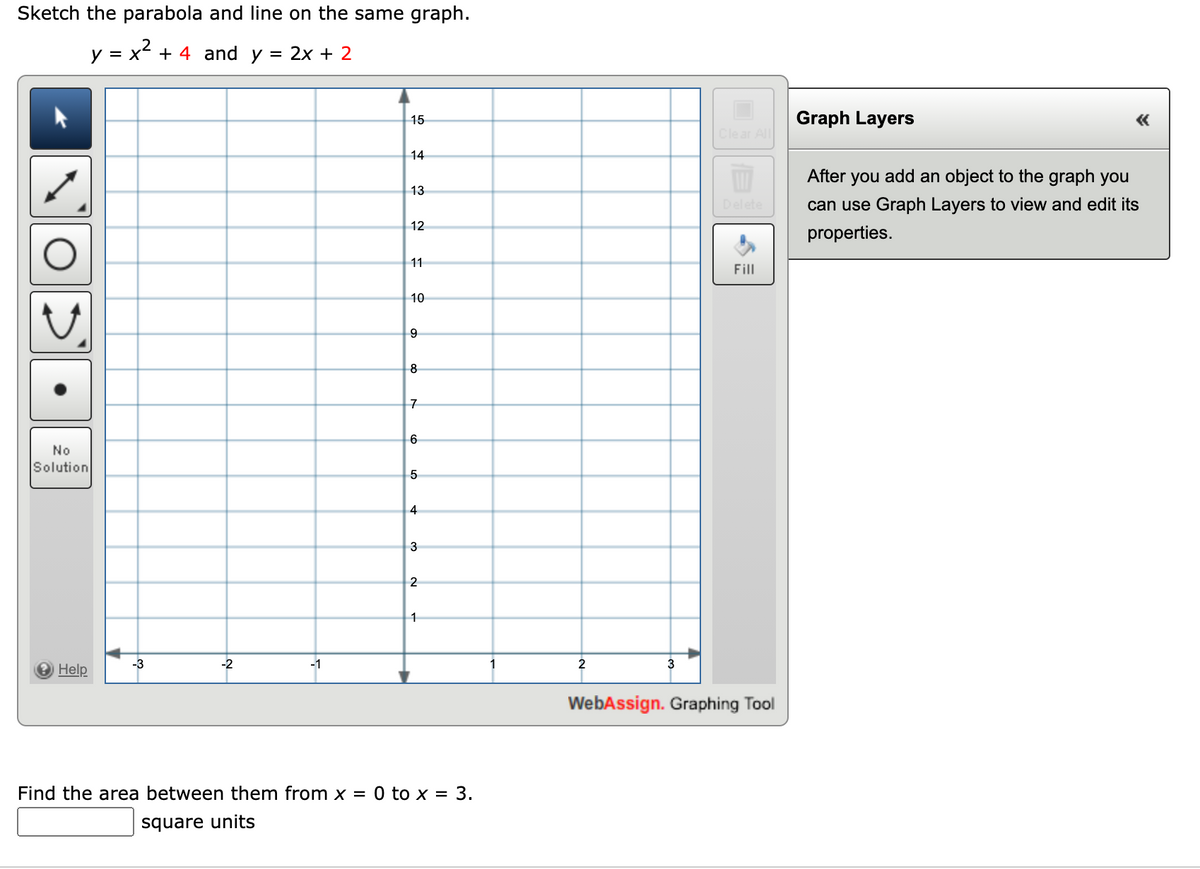



Answered Sketch The Parabola And Line On The Bartleby



1



Solution Sketch The Graph Of F X 2x 2 8x 11 It Is A Parabola With Its Lowest Vertex In Which Quadrant Use Window Of 10 To 10 Both X And Y



Quadratic Graph Example Y Ax C Expii



What S The Axis Of Symetry Vertex And Graph Y 2x 2 6x 1 Mathskey Com




Y 2x 2 Parabola Novocom Top



View Question The Parabolas Defined By The Equations Y 2x 2 4x 4 And Y X 2 2x 4 Intersect At Points A B And C D Where C Ge A What Is C A



Solution Find The Vertex Of The Parabola Y 2x2 12x 13



Y 2x 2 Youtube



01da Quick Graph Y 2x 2 Youtube



Organize The Information For Your Parabola Graph Your Parabola Y 2x 2 12x 19 Axis Of Symmetry Snapsolve




6 The Graph Of The Parabola Given By The Function Y 2x2 2x 1 Opens Up Or Down Incorrect Your Answer Down Correct Answer Down Explanation The 2 In Y 2x2 2x 1




Characteristics Of Parabolas College Algebra



Quadratics Graphing Parabolas Sparknotes
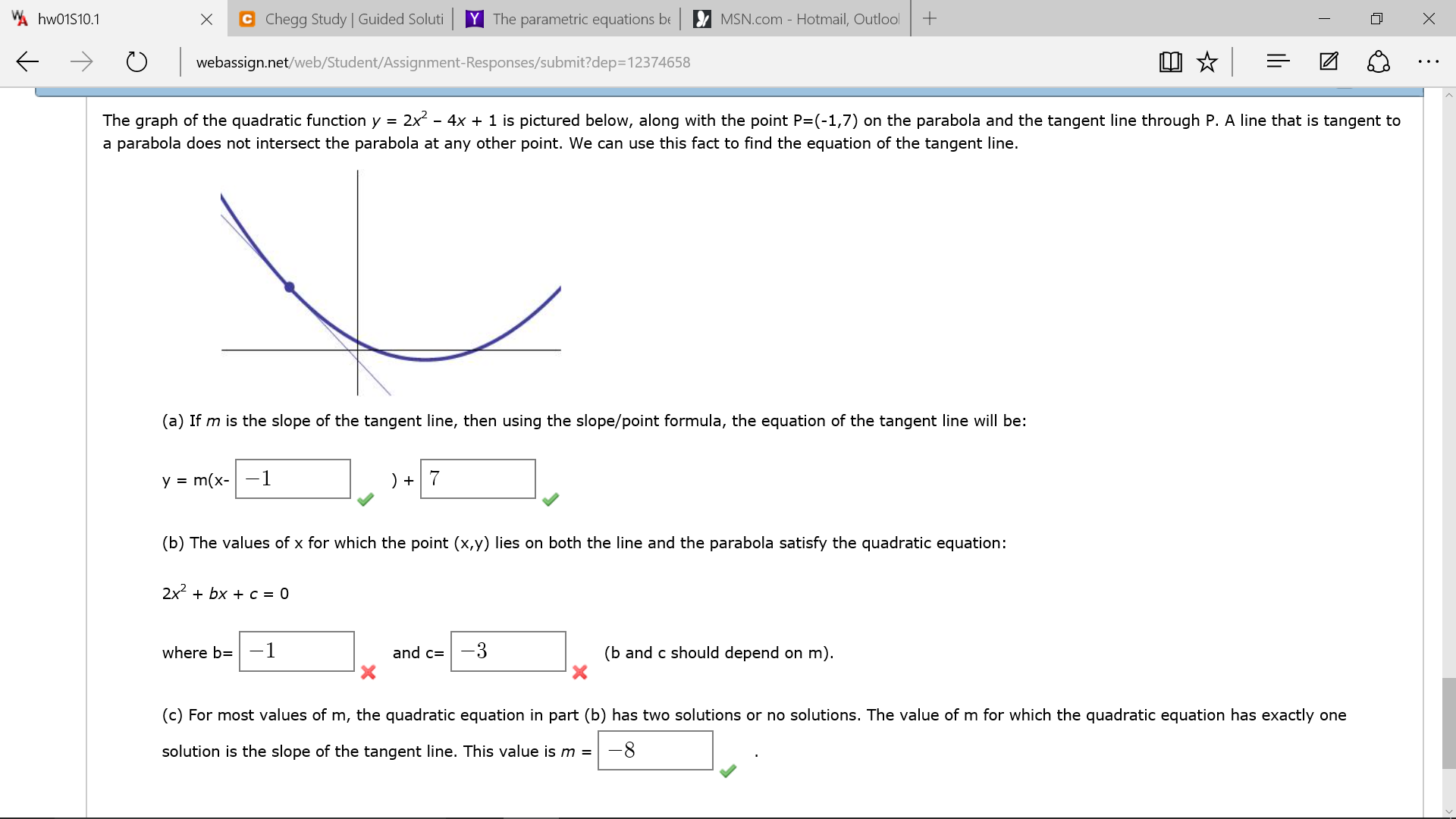



The Graph Of The Quadratic Function Y 2x 2 4x 1 Chegg Com



Quadratic Graph Example Y Ax Expii




Vertex Axis Of Symmetry Of A Parabola Video Khan Academy



Quadratics Graphing Parabolas Sparknotes




Transformations Of Quadratic Functions College Algebra




Find The Area Enclosed By The Parabolas Y 5x2 And Y 2x2 9 Mathematics Shaalaa Com



Parabolas And Cubics
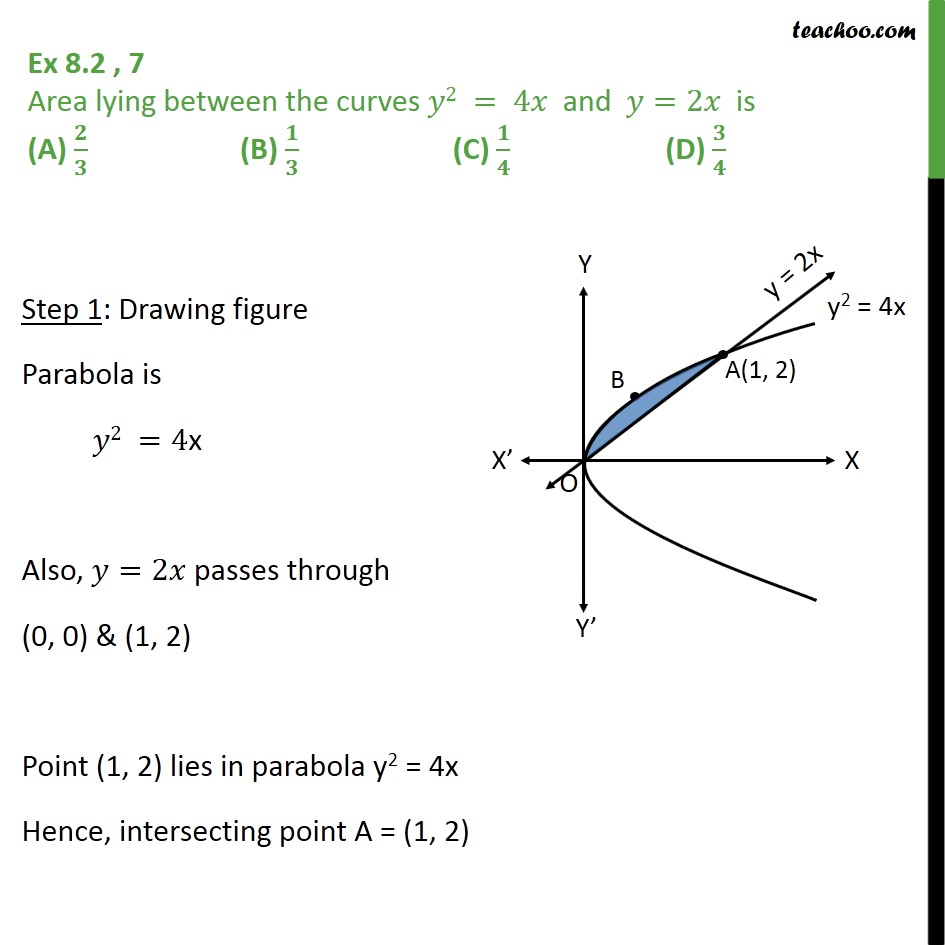



Ex 8 2 7 Area Lying Between Y2 4x And Y 2x Is Ex 8 2



Quadratic Function Parabola




How To Draw Y 2 X 2



Draw The Graph Of Y 2x 2 3x 5 And Hence Solve 2x 2 4x 6 0 Y 2x 2 3x 5 Sarthaks Econnect Largest Online Education Community




Y 1 2x 2 Parabola Novocom Top



1
:max_bytes(150000):strip_icc()/1000px-Parabola_features-58fc9dfd5f9b581d595b886e.png)



Quadratic Function Changes In The Parabola



Parabolas Xcelerate Math




Graph Of Y X 2 The Equation For A Parabola Download Scientific Diagram




What Is The Area Enclosed By The Parabola Y2 2x And The Line Y 2x Quora



1



How To Do You Graph Y 2x 2 X 3 By Plotting Points Socratic



Exploration Of Parabolas



Parabolas
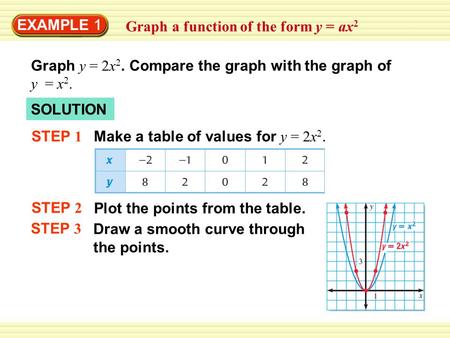



Example 1 Graph A Function Of The Form Y Ax 2 Graph Y 2x 2 Compare The Graph With The Graph Of Y X 2 Solution Step 1 Make A Table Of Values For Ppt Download




Parabola Sketching Y 2x 2 4x 6 From The Parabola Translation Download Scientific Diagram




Q15 The Area Common To The Parabola Y 2x2 And Y X2 4 Is



Content Transformations Of The Parabola
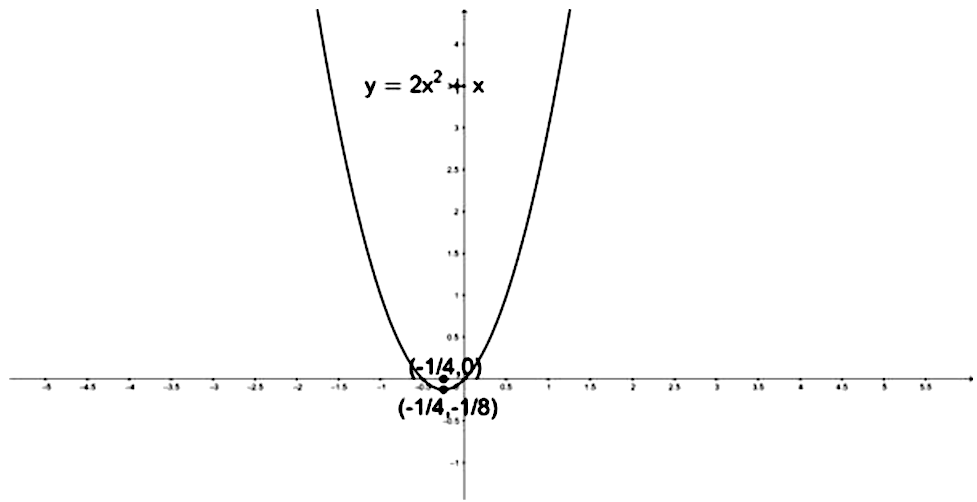



The Focus Of The Parabola Y 2x 2 X Is A 0 0 B 1 2 1 4 C 1 4 0 D 1 4 1 8 Snapsolve




Please Help Asap Correct Answer Only Please The Graph Of The Function Y X2 Is Shown How Brainly Com



Instructional Unit The Parabola Day 4 And 5
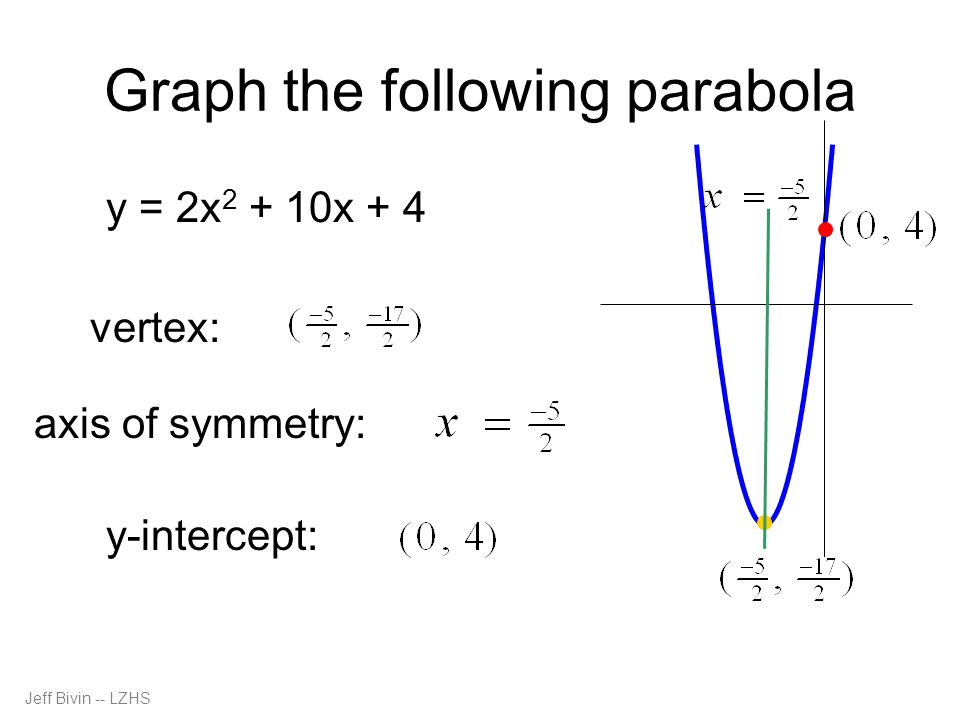



Graphing Parabolas Using The Vertex Axis Of Symmetry Y Intercept By Jeffrey Bivin Lake Zurich High School Last Updated October Ppt Download
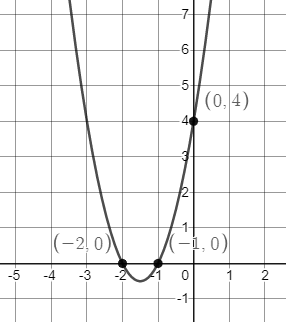



Sketch The Parabola Math Y 2x 2 6x 4 Math By Using Its Intercepts Homework Help And Answers Slader



Graphing Quadratic Functions



2



Quadratic Function Parabola




How To Graph A Parabola 13 Steps With Pictures Wikihow



The Line Y 2x 9 Is Tangent To The Parabola Y X 2 Ax B At The Point 4 1 What Are The Values Of A And B Quora




Is The Parabola Described By Y 2x 2 Wider Or Narrower Than The Parabola Described By Y X 2 Socratic
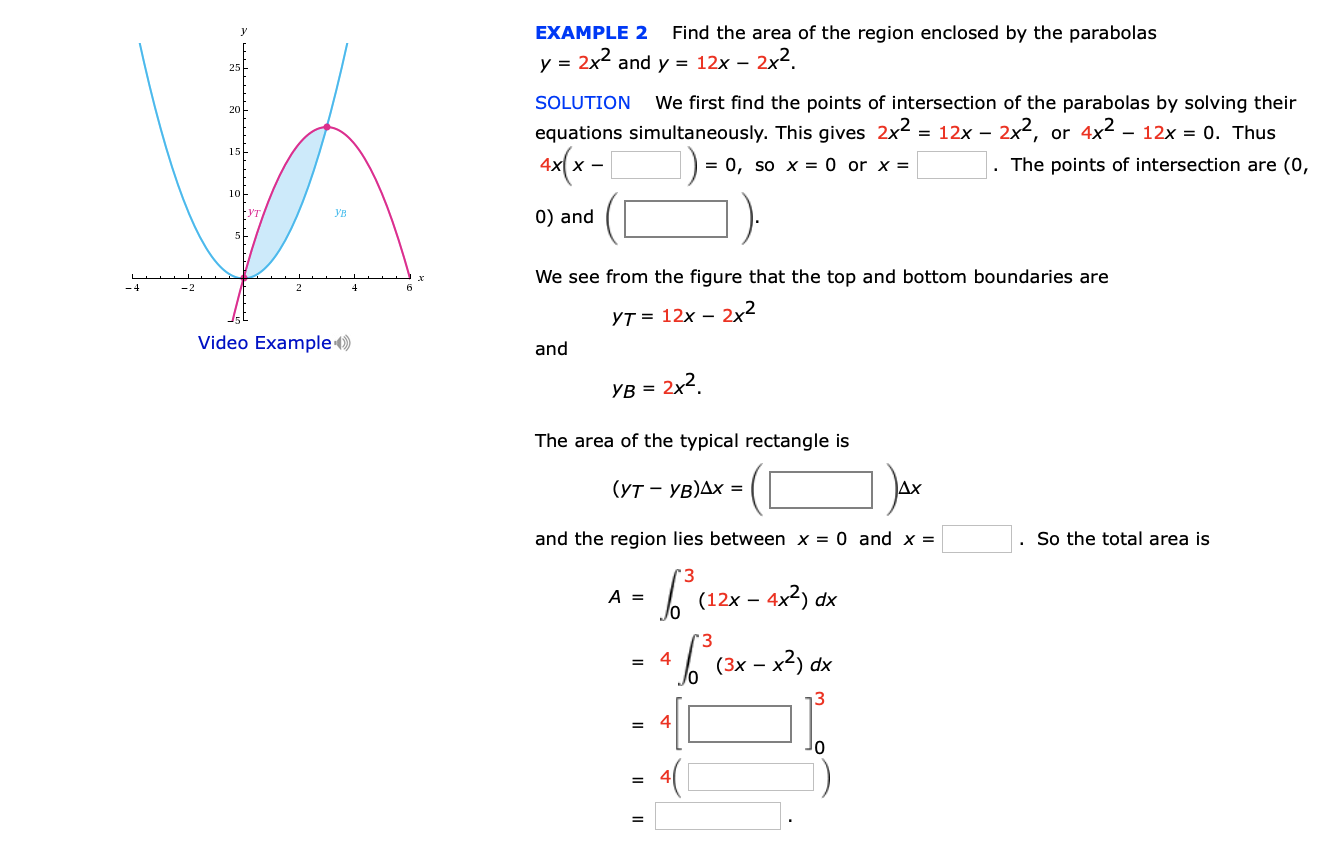



Find The Area Of The Region Enclosed By Chegg Com



0 件のコメント:
コメントを投稿